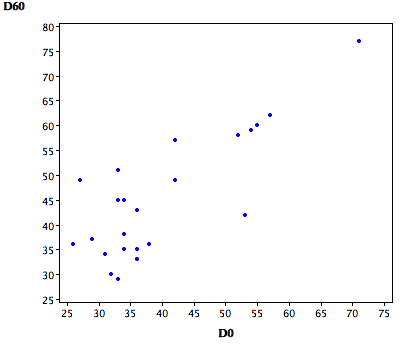
Consider the data from this website.
They present the attached graph and state
"It is clear that children who get more correct in the D0 condition tend to get more correct in the D60 condition. The correlation between the two conditions is high: r = 0.80. Clearly these two variables are not independent."
Why does correlation here mean that independence is violated?
The definition of independence on wikipedia says:
"two random variables are independent if the realization of one does not affect the probability distribution of the other."
I don't see how the presence of correlation implies that one variable affects the probability distribution of the other.
I mean, we can ASSUME that one variable being high affects the probability of the other variable being high. But here they are not stating it as an assumption but as a truth.
Why can it not be the case that there is some individual-specific factor at play? For example, Maybe certain subjects have a lot more probability mass on the right tail. This would give correlation, but I don't think it means that one variable being large affects the probability of the other variable being large
(Aside: To whoever upvoted this prior to the major edit, my apologies if I have inadvertently changed what you were most interested in)