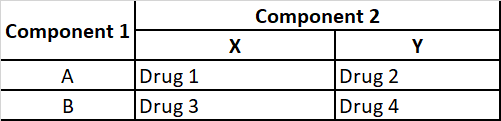
I'm going to analyze the effect of four drugs on a continuous outcome. Each drug has a specific combination of two components with two levels each, i.e. there's one drug for each combination. My question is: Is it appropriate to analyze the components as two independent variables (with two levels each) or do I have use a single IV with four levels?
If I understand correctly what you want to do, the components here are rather factors than variables, and what you call "independence" refers to "absence of interactions", i.e., it is not of interest whether they are "stochastically independent" as random variables (because you will actually choose whatever combination of them as part of your design), but rather whether the effect of each component on your outcome depends on the level of the other component, which is called interaction. In a standard analysis of such data, you would treat the components as two factors with two levels each, and then from your data you can test presence or absence of such interactions.
In any case, whether there are interactions or not, the standard analysis will also allow you to estimate the mean outcome of all four combinations.
What I meant was that while any combination is possible and all combinations exist, the drugs aren't all equally common either in the population or the sample. That means some combinations are more common so one could argue (perhaps erroneously) that the level of one component affects the probability of each level of the other.
The effect of each component and whether there is an interaction effect on the outcome is exactly what I want to test. If that's not appropriate, I will instead use the variable drug as an IV with 4 levels.
