2
$\begingroup$

can anyone help with this question

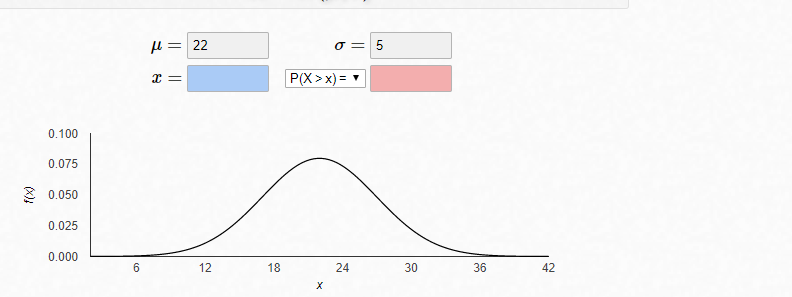
Q: According to a survey of 10000 students, the average distance to the university is 22 minutes with a standard deviation of 5 minutes (normally distributed). a) Plot (sketch) the distribution including all relevant labels. b) How likely is it that a randomly sampled student has a distance between 15 and 25 minutes? c) How large does the interval need to be, so that a randomly samples student belongs to the 95% (99%) of the most probable distances?

so far i have done sol:: i have plotted a graph that ie most likely to fall under the 95 % if we standardize the sample mean then we can directly see in which probability area in which it lies let say we have a z score 2.5 it outside of the most unlikely events under the distribution this would called significance unit

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Add the self-study tag and read the tag wiki. $\endgroup$
    – StubbornAtom
    Commented May 25, 2020 at 7:51

1 Answer 1

Reset to default
2
$\begingroup$

This would be your chart

For the second question just do:

  • The cumulative distribution until 25 minus the cumulative distribution until 15. So answer would be 64,5%

For the last question you need to know that on a normal distribution 95% of the values are within 2 standard deviations and 99,7% of the cases are withing 3 standard deviations.

Improve this answer
$\endgroup$
1
  • $\begingroup$ thank u so much $\endgroup$
    – user286249
    Commented May 25, 2020 at 6:11