What do we commonly call a Sampler ? and the link between MonteCarlo, Metropolis-Hasting method, MCMC method and Fisher formalism

Question

Sorry to ask multiples questions but they are all related to the same problematic. I would like to get explanations/clarifications as much as possible since I am going to use all these methods in the context of a Master degree.

1) I make confusions between what we call a "sampler". From what I understand, a sampler allows to generate a distribution of points that follows a known PDF (probability distribution function), doesn't it ?

2) On the other side, it exits Monte-Carlo method which allows for example to compute the number Pi by generating random values (x_i,y_i) and see if x_i^2+y_i^2 < R^2. The ratio between accepted points and total points generated will an estimation of Pi.

3) Moreover, I have used previously the Metropolis-Hasting in this simple form, i.e to generate a distribution of points with a known PDF. But I have also seen that we can use it to do estimation of parameters of a model : at which level can we distinguish the "Sampler" Metroplis-Hasting from "Estimation of parameters" method.

4) For example, there is also the acceptance method (called also Von Neumann method), very used in Nuclear physics, which generates also a distribution from a known PDF : can it be qualified also of "sampler" ?

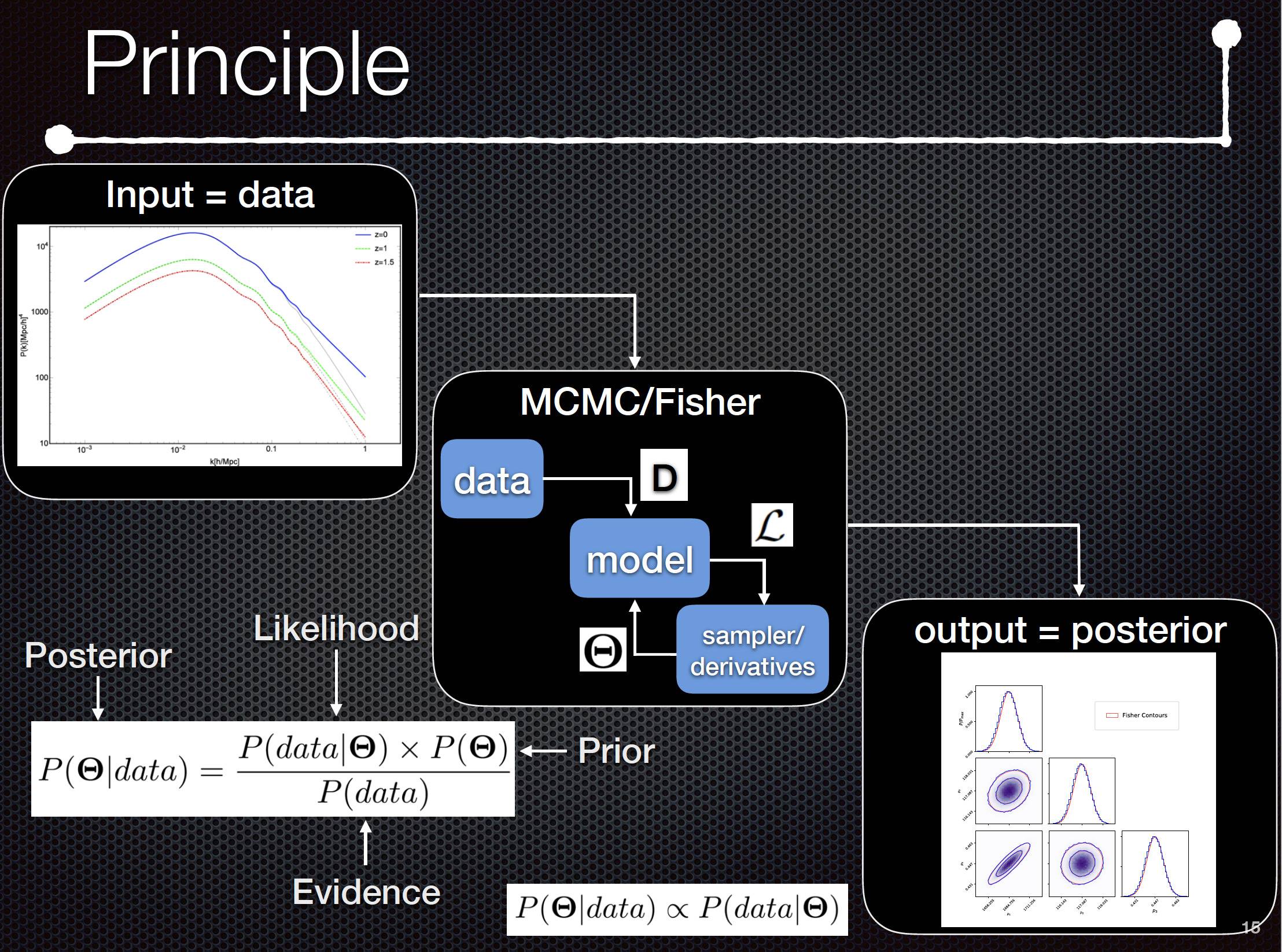
5) Finally, the Markov chain coupled with Monte Carlo (MCMC) is a pure method to estimate the parameters of a model given the data: so IMPORTANT QUESTION : What is the respective role of Monte_Carlo and the Markov chain in this method ?

To summarize, I show you below the problematic in which I am: it is about Forecast in astrophysics. In this post, I am talking about "Inverse problem" in Physics, i.e, we don't predict the data from a very accurate theoretical model but I want to estimate the parameters of my theoretical model given the data I have from experiment or from simulated data (what's we call fake data). The using of Bayes theorem is very practical in this kind of approach since we have a relation of proportionality between the posterior (probability of parameters given the data) and the likelihood (product of PDF taken at data values given the parameters model).

6) Fisher formalism is very useful for estimation of the standard deviation compared to the fiducial values but we need to know these fiducial values before and second point, we have to assume that posterior distribution is always Gaussian, haven't we ? (or that likelihood is Gaussian, I don't remember ... if someone could indicate this assumption).

So as you have seen, there are a lot of concept to integrate for me and I would like to convert this mess into ordered things.

First, I would like to make the difference between a "sampler" and an estimator method. After, any remark is welcome to clarify my confusions.

Remark: I have asked different questions but all of them is linked in the sense they use random processes to generate distribution or evaluate parameters (by the Bayes theorem).

Answer 1

Let's clear things up. Metropolis-Hastings is an Markov chain Monte Carlo (MCMC) algorithm. "Monte Carlo" in MCMC stands for "simulation" (though there are non-Monte Carlo simulation algorithms). MCMC algorithms are used to sample, not estimate, values from the distributions. Having samples from the distributions, we can use some sample statistics, e.g. sample mean, to calculate things like mean of the distribution, and treat this result as a point estimate. "Sampler" is an algorithm, so a Monte Carlo algorithm, that is able to sample, though I don't recall seeing this term often in the academic literature, but rather as a technical term used in software documentation.

I'm not sure if I follow what you mean by "Fisher formalism", but it seems that you are talking about Laplace approximation, i.e. method to approximate the posterior distribution with a Gaussian (rather then sample directly from the distribution, as in MCMC). We use it usually when MCMC is computationally too expensive, so we cannot afford it. There are other algorithms to approximate posterior distributions as well, Laplace approximation is just one of the examples.