Confusion related to derivation of the log likelihood

Question

I was reading the Hastie, Friedman, Tibshirani paper "Sparse Inverse Covariance Estimation with the Graphical Lasso" and it had the following

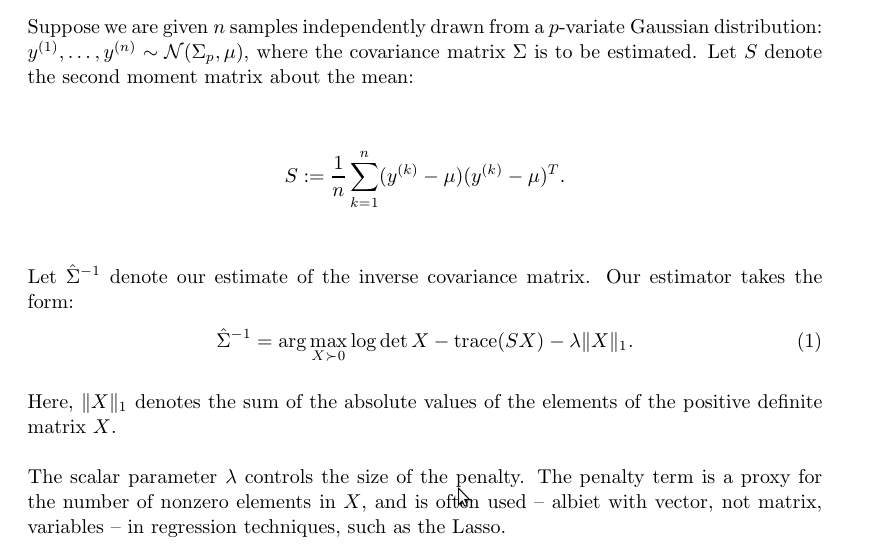
I couldn't get how the following expression was derived

$\hat\Sigma^{-1} = \displaystyle\arg \max_{X \succ 0} \log \mbox{det}X - \mbox{trace}(SX) - \lambda||X||_1$

I know that they are calculating the likelihood of the data and maximizing with respect to the mean $\mu$ as given in the paper and it results in the expression above. How is the expression obtained?

Answer 1

This result can be obtained by writing the log likelihood of the multivariate gaussian distribution and using the property that, for a square matrix $A$ and a vector $x$ of same size, $x^TAx=tr(Axx^T)$. The extra term $-\lambda||X||_1$ does not come from the likelihood, it is there to regularize the problem.