Consider a circle with a circumference of $n$. On this circle, I define two arcs of length $k<n$, $A_1$ and $A_2$. The centres of the two arcs are uniformly distributed on the circle.
Let $\Omega_{1}=A_1 \setminus A_2$ and $\Omega_{2}=A_2 \setminus A_1$ such that the length of $\Omega_1 \cup \Omega_2$ is $2k$ minus the overlapping part of the two arcs.
What is the expectation of the length of $\Omega_1 \cup \Omega_2$?
Let's generalize a bit and suppose the arcs may have distinct lengths $|\Omega_i| = k_i.$ Without any loss of generality, suppose $k_1 \le k_2.$ Establish a coordinate system on the circle in which the arcs are oriented positively and $\Omega_2$ begins at 0. Let $x$ be the point at which $\Omega_1$ begins: see the figure.
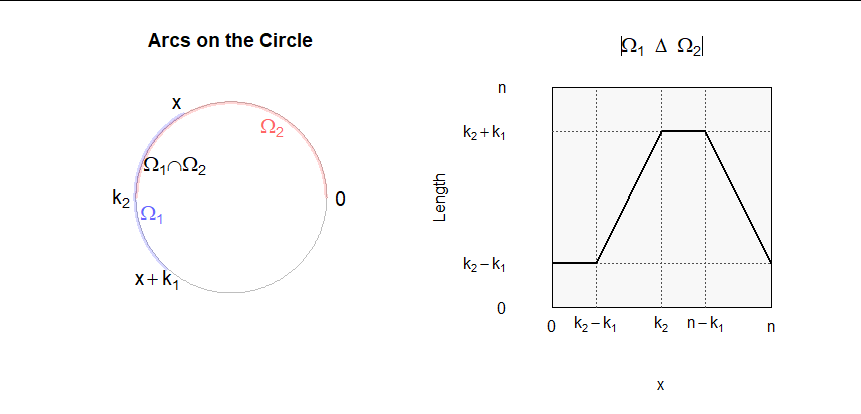
When $x=0,$ $\Omega_1\subset\Omega_2$ and the length of their symmetric difference $\Omega_1\Delta\Omega_2 = \left(\Omega_1\setminus \Omega_2\right) \cup \left(\Omega_2\setminus\Omega_1\right)$ is $k_2-k_1.$ As $x$ increases, this length remains the same until the terminus of $\Omega_1$ just sticks beyond the terminus of $\Omega_2,$ when $x=k_2-k_1.$ At that point the length of the difference increases linearly until the arcs are disjoint, where $x=k_2$ and the length is $k_1+k_2.$
In the figure, $k_1+k_2\le n,$ implying the arcs do not necessarily overlap. Thus, until $x=n-k_1,$ the length remains at $k_1+k_2.$ From that point until $x=n,$ the length decreases linearly back to $k_2-k_1.$
The right hand figure plots the length as a function $X$ of $x$ in the case $k_1+k_2\le n.$ Let's analyze this case (leaving the case $k_1+k_2\gt n$ as an exercise).
The location of $x$ has a uniform distribution with density $1/n.$ (This is an obvious consequence of the independence of the arc locations and the uniform distribution of the location of $\Omega_1.$) By definition, the expectation of the length is
$$E[X] = \int_0^n X(x)\,\frac{1}{n}\,\mathrm{d}x = k_1 + k_2 - \frac{2k_1k_2}{n}.$$
(This is most easily evaluated by elementary geometry, because it is $1/n$ times the area under the curve.)
Remember, this solution applies only when $k_1+k_2\le n.$
