How to predict the standard deviation that is changing over time?

Question

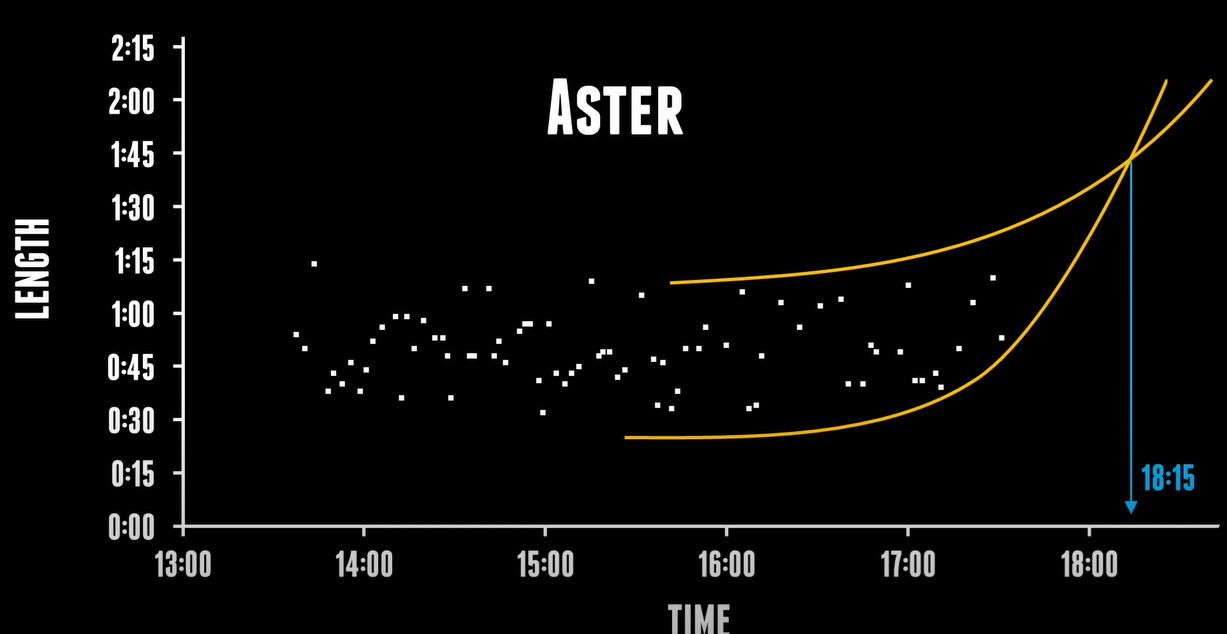
I just saw this interesting video regarding the prediction of child birth based on the regularity and duration of one's contraction. Now what I do not fully understand is how one can predict when the standard deviation reaches zero. I thought most linear regressions do not attempt to predict the standard deviations in the future. I feel like I am missing something fundamental with respect to regression as I cannot seem to figure out how one does this.

Please see the figure below to see what I mean.

Could someone explain the technique in predicting when the envelopes (lower and upper bound) of the predicted standard deviation reaches zero? And if possible, how this translates to possible predicted data points?

Thanks!

Answer 1

when you have a useful model the residuals should have constant variance i.e. not dependent on level and not autoregressive in nature. Thus the residuals need to have homogenous error variance. If the residuals have a predictable variance then one needs to either segment the data ala the CHOW test or to determine a suitable power transform via a BOX-COX power transform.

If you wish you can post an actual time series and I might be able to help further.

After a visual review of the data plot it appears that that the model error variance has increased over time BUT only an analysis of the original data could test that hypothesis. The simple variance of the observed series i.e. a simple mean model appears to have dampened BUT what is of concern is the error variance from a useful auto-projective model or a deterministic model containing trends or level shifts.