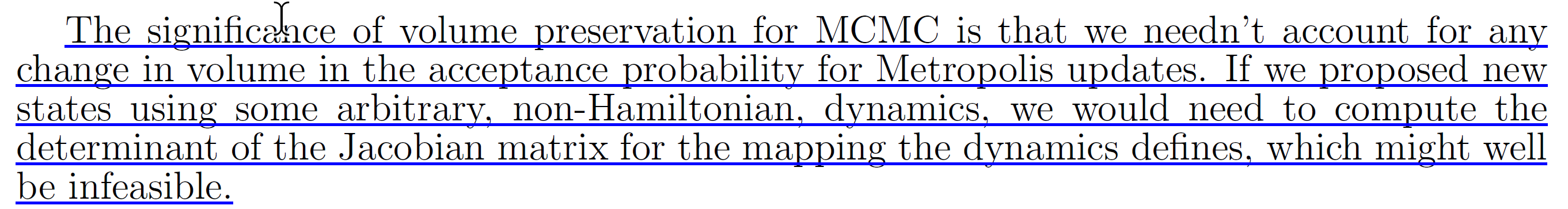
In the paper of MCMC using Hamiltonian dynamics, there is the following statement on volume preservation. What does it mean exactly? I am not very clear about the relationship between volume and acceptance probability.
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Better late than never. I also thought about this question when I first came across the paper, and my self-given answer is the following. In MCMC, if we are at $x$ and we get at $y$ what we want to calculate is $\frac{p(y)}{p(x)}$. Both these are pdf implicitly assumed to be a pdf with respect to the Lebesgue measure(the standard measure of R^d). Now, if I make a non-volume preserving transformation, I would not have anymore a pdf with respect to the Lebesgue measure for ${y}$, hence the $p(y)$ above is not a pdf anymore, and the procedure would not work. Before making the ratio hence, I would have to adjust for this to get back a pdf with respect to the Lebesgue measure. This can be very painfull, as I would need to calculate the Jacobian and then its determinant, which can be computationally very heavy.
