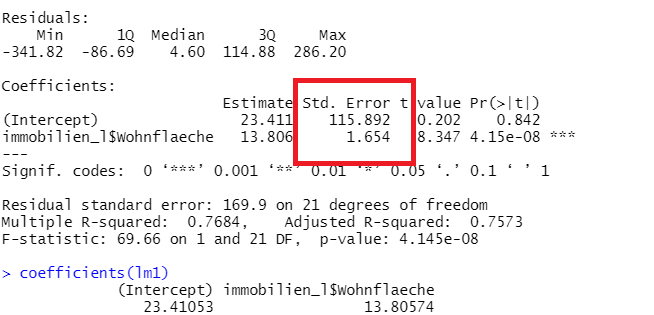
Can you help me to interpret the following standard deviation? It is a linear regression problem, I need to predict Rent which depends on the size of an Apartment. Rent=23.411+13.806. The question is how to interpret the standard errors 115.892 and 1.654. I know how to calculate them
1 Answer
The standard error it's a metric that show the deviation that your estimated coefficient could have from the estimated value (mean), that is, the error. It's a number in the same units as the coefficient, which could also be multiplied by respective variable to be read in terms of the dependent variable if needed.
It helps you create the confidence intervals, depending on your level of confidence (usually it's 95%) which helps you understand the range of values that the unknown parameter can actually have.
If your variable is size, then the value of 1.654 means that the estimated coefficient has mean 13.806 but could be between 12.152 (13.806-1.654) and 15.46 (13.806+1.654) for example, which corresponds to a confidence level of 68%. Same interpretation goes for the intercept. So the impact of size could be between 12.152 and 15.46 towards the Rent.
If you want a greater confidence level, say 95%, you need to (basically) multiply the interval by two, that is: 10.498 (13.806-1.654 x 2) and 17.114 (13.806+1.654 x 2) respectively.
-
2$\begingroup$ The units of the coefficients are never those of the dependent variable except when the corresponding explanatory variable is unitless. In this case the latter can be roughly thought of as surface area ("living space"), so its coefficient is rental cost per unit area of living space, not the rent itself. $\endgroup$– whuber ♦Commented May 24, 2022 at 22:29
-
$\begingroup$ Re the edit: it's still incorrect. The standard error must be in the same units as the coefficient itself, not the dependent variable! Otherwise, none of your arithmetic operations (such as adding and subtracting multiples of the SE from the coefficient estimate) would make any sense. $\endgroup$– whuber ♦Commented May 25, 2022 at 14:30