Transform bivariate uniform variable

Question

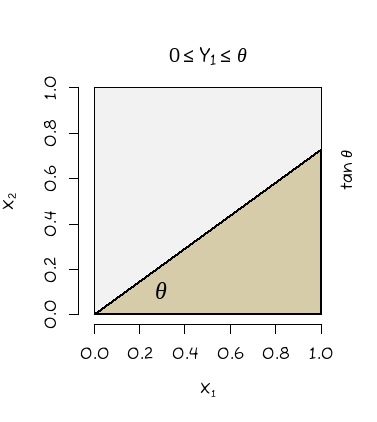
Let $X_1 = U(0,1)$ and $X_2 = U(0,1)$. $X_1$ and $X_2$ are independent. Then $f(x_{1}, x_{2})=1, {0}\le{x_1}\le{1}, {0}\le{x_2}\le{1}$.

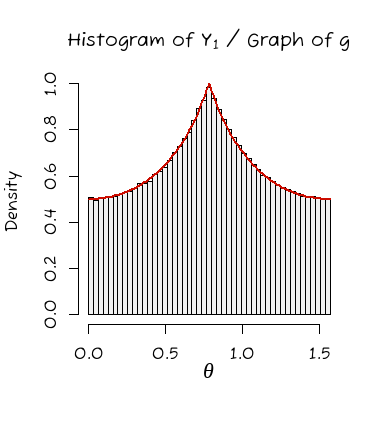
Let $Y_1 = \arctan(X_{2}/X_{1})$, $Y_2 = X_2$. I need to find the density function $g(y_1)$.

Here's what I've done so far.

$X_{2}/X_{1} = \tan(Y_{1})$ $X_{1} = X_{2} / \tan(Y_{1}) = X_{2}\cot(Y_{1})$

Since $X_{2}=Y_{2}$, $X_{1}=Y_{2}\cot(Y_{1})$.

The Jacobian of the transformation is give by the matrix $J = \begin{bmatrix} \frac{\partial{y_2\cot(y_1)}}{\partial{y_1}} & \frac{\partial{y_2\cot(y_1)}}{\partial{y_2}} \\ \frac{\partial{y_2}}{y_1} & \frac{y_2}{y_2} \end{bmatrix} = \begin{bmatrix} -y_{2}\csc^{2}(y_{1}) & \cot(y_1) \\ 0 & 1 \end{bmatrix}$. So, $\det(J)= -y_{2}\csc^{2}(y_{1})$.

At this point, I am kinda lost. I know that I need to compute $g(y_1, y_2)$, find its domain, and then integrate with respect to $y_2$. I do not understand how to find the joint density $g(y_1, y_2)$, could you please help?

Answer 1

Find the distribution function of $Y_1$ with a picture, then differentiate it.

Observe that because $(X_1,X_2)$ lies in the first quadrant and $Y_1$ is the angle subtended by this point, $0\le Y_1 \le \pi/2.$ Moreover, for any possible value $\theta$ in this interval, the event $Y_1 \le \theta$ is the region in the unit square bounded above by the line at angle $\theta,$ highlighted in this diagram:

Because $(X_1,X_2)$ is uniformly distributed on the unit square, the probability of any event equals its area.

Clearly when $0\le \theta\le \pi/4,$ where this event is a triangle, its area is half its height:

$$\Pr(Y_1 \le \theta) = \frac{1}{2}\tan\theta, \quad \theta \le \pi/4.$$

Its derivative is $\sec^2(\theta)/2.$

When $\pi/4\le\theta\le \pi/2,$ the event is the complement of a triangle, immediately giving

$$\Pr(Y_1 \le \theta) = 1 - \frac{1}{2}\cot\theta, \quad \pi/4 \le \theta \le \pi/2.$$

Its derivative is $\csc^2(\theta)/2 = \sec^2(\pi/2-\theta)/2.$

Combining these results into a common formula gives

$$g(\theta) = \frac{1}{2}\sec\left(\min\left(\theta, \frac{\pi}{2}-\theta\right)\right)^2,\quad 0\le\theta\le\frac{\pi}{2}.$$

Of course $g\equiv 0$ for all other arguments.

To illustrate $g,$ here is a histogram of a million realizations of $Y_1$ (computed with the R code n <- 1e6; y <- atan2(x2 <- runif(n), x1 <- runif(n))) over which the graph of $g$ is plotted in red.

Answer 2

The transformation from $(x_1,x_2)$ to $(y_1,y_2)$ is given by $h(x_1,x_2) = (h_1(x_1,x_2),h_2(x_1,x_2))^t = \left(\arctan(\frac{x_2}{x_1}), x_2\right)^t$. The jacobian matrix $J(x_1,x_2)$ is given by:

$$ J(x_1,x_2) = \begin{bmatrix} \frac{\partial h_1}{\partial x_1} & \frac{\partial h_1}{\partial x_2} \\ \frac{\partial h_2}{\partial x_1} & \frac{\partial h_1}{\partial x_2} \end{bmatrix} = \begin{bmatrix} \frac{-x_2}{x_1^2 + x_2^2} & \frac{x_1}{x_1^2 + x_2^2} \\ 0 & 1 \end{bmatrix}, $$

with the absolute value of the determinant $|J(x_1,x_2)| = \frac{x_2}{x_1^2 + x_2^2}$.

As mentioned by Xi'an in the comments, you can find the density probability function $g(y_1,y_2)$ of $(Y_1,Y_2)$ with the following formula: $$ g(y_1,y_2) = \frac{f(x_1,x_2)}{|J(x_1,x_2)|}|_{(x_1,x_2) = h^{-1}(y_1,y_2)} = \frac{x_1^2 + x_2^2}{x_2}|_{(x_1,x_2) = h^{-1}(y_1,y_2)}, $$

$$ = \frac{y_2^2\cot(y_1)^2 + y_2^2}{y_2} = y_2(\cot(y_1)^2 + 1), $$

where $f(x_1,x_2)$ is the probability density function of $(X_1,X_2)$. Note that this works only for bijective transformations (mapping) $h(x_1,x_2)$.