What are we testing in measurement invariance?
The first theorem of Meredith (1993) (p. 528) states that a random variable $X$ is
measurement invariant with regards to selection on $V$ if and only if they are locally independent when conditioned on $\eta$, for every $\eta$ in the sample space.
Consider now that $X$ are your depression scale items, $\eta$ is your latent depression construct score and $V$ is sex. What measurement invariance implies is that, in the diagram $V \rightarrow \eta \rightarrow X$, conditioning on the mediator $\eta$ should block any effect that $V$ could have on $X$, i.e. there is no direct effect from $V$ to $X$. The only way that sex ($V$) can influence depression item scores ($X$) is through a difference in the latent construct score ($\eta$).
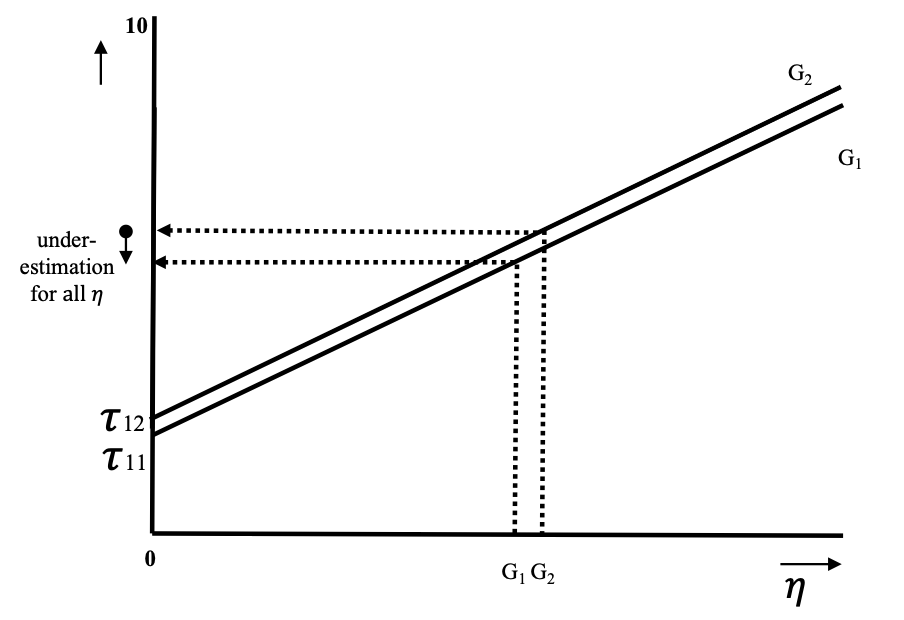
In scalar invariance (or strong factorial invariance), both the slopes of the effect of the latent common scores on the item scores and the item intercepts are assumed equal across groups. Under this assumption, if girls report higher average scores in the observed items $X$, it must be due to higher average latent depression scores $\eta$.
Your question
Note that you incorrectly state
This does not hold for my analysis. Because girls report more depression, their factor intercepts are higher. Thus, this measure fails the measurement invariance test.
Girls having higher latent factor intercepts does not violate measurement invariance nor scalar invariance. As we saw in the diagram, there is no problem with sex influencing directly on the latent factor score ($V \rightarrow \eta$). The issue is when sex would influence the item scores directly without reflecting a change in the latent factor ($V \rightarrow X$).
Then, your question is no longer a statistical one, but at least a psychological one if not an epistemological one. What you would like to know is whether the average increase in item scores for girls is reflecting an increase in actual latent depression score, or just a difference in social desirability for reporting in contrast to boys. Also note that you say that girls over-report depression, which already reflects some unwarranted implicit assumptions (i.e. it is not boys that under-report depression).
Without further assumptions which would strongly depend on domain knowledge (that might not even exist), you cannot turn this into a testable statistical problem. For instance, what specific functional form would you expect for the effect of desirability on reporting? Should it affect all sub-scales or items in the same way? Would only a subgroup of girls be affected? etc.