Is there a similar way to this for calculating the $\alpha$ and $\beta$ parameters for a Beta distribution if I know the harmonic mean and variance that I want the distribution to have ($\alpha>1$ and $\beta>0$)?
$HM=\frac{\alpha-1}{\alpha+\beta-1}$
Example: harmonic mean = 0.25 and variance = 0.04 should yield $\alpha$ = 2 and $\beta$ = 3
As per JimB’s comments below; is there a single solution if $\alpha > 1$ and $\beta > 1$?
You are not guaranteed a single solution.
If you do the substitutions as suggested by whuber, you will end up having to solve a cubic expression for $\alpha$ or $\beta$ which with minor difficulty will lead to three pairs of solutions.
In your example, you will get $\alpha=1+\frac\beta 3$ and something like $$64\beta^3-33\beta^2-495\beta+54 =0$$ and solutions $$(3,2) \text{ and }\\ \left(\frac{9 \sqrt{41}+75}{128},\frac{27 \sqrt{41}-159}{128}\right) \text{ and } \\\left(\frac{-9 \sqrt{41}+75}{128},\frac{-27 \sqrt{41}-159}{128}\right) $$ where you can reject the third pair as it has $0< \alpha<1$ and $\beta<0$.
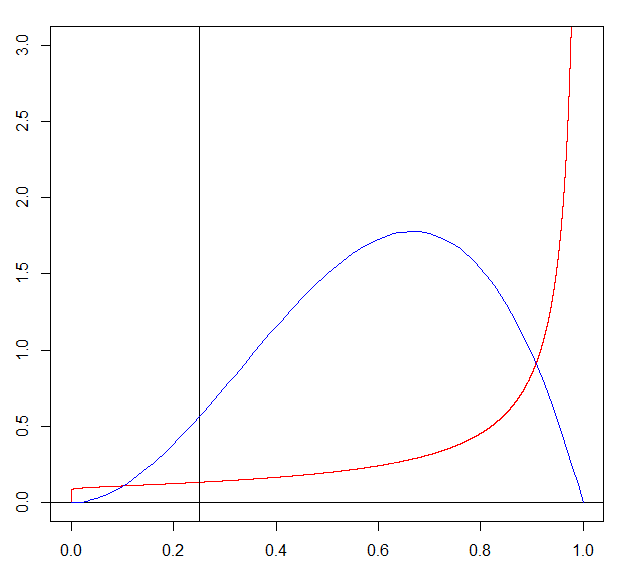
The second pair with $\alpha \approx 1.036157172944497$ and $\beta\approx 0.1084715188334915$ appears to be a solution satisfying the conditions. It is a deeply strange solution as $61\%$ of the probability would be concentrated in the interval $[0.99,1]$ and less than $3\%$ below the harmonic mean, and this raises the question of whether the harmonic mean is an useful statistic for describing a Beta distributed sample.
This shows the probability densities of the two solutions - the first in blue and the second in red, with the harmonic mean as a vertical black line. It is the small but substantial probability of extremely small values using the second solution which brings its harmonic mean down, despite the vast bulk of the distribution being much higher (its pdf is unbounded near $1$).
