Is this a typo on P.75, Theorem 5.52 of the book "Asymptotic Statistics" by Van der Vaart?

Question

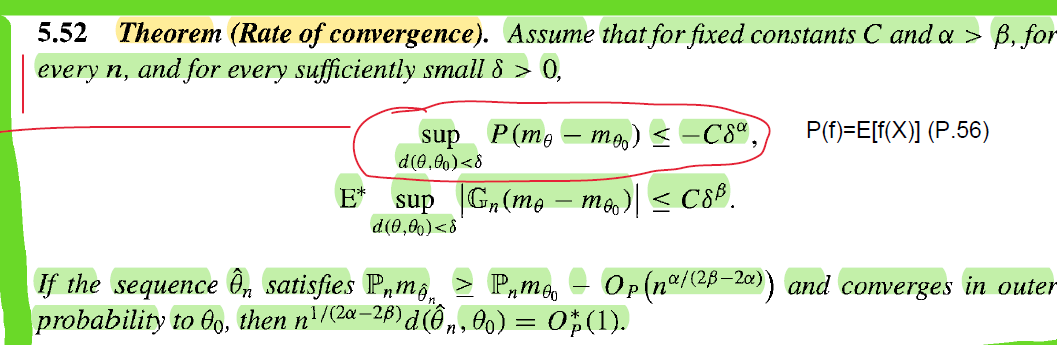
Let $\Theta$ be a compact metric space, $\theta \in \Theta.$ Let $m_{\theta}:\mathbb{R}^d\to \mathbb{R}: x\mapsto m_{\theta}(x)$ be a family of measurable function indexed by $\theta \in \Theta.$ Let $P(f):=E[f(X)],$ where $X:\Omega \to \mathbb{R}^d, f:\mathbb{R}^d\to \mathbb{R}.$ So below, $P(m_{\theta}-m_{\theta_0})$ should be $E[m_{\theta}(X)-m_{\theta_0}(X)].$ Also below:

$$\theta_0:= arg max_{\theta \in \Theta} P(m_\theta(X))= arg max_{\theta \in \Theta} E[m_{\theta}(X)].$$

I'm having to look into a few results in M-estimation from the book "Asymptotic Statistics (2000)" by Van der Vaart, and I'm looking at this theorem on P.75: (I think) here $\theta_0$ is a value of the parameter $\theta$ that maximizes the maximum likelihood estimate. Below,

It seems to me that sup in the first line is a typo, as the quantities on the LHS there are nonpositive and bounded by something strictly negative, however, at $\theta=\theta_0,$ the quantities on the LHS achieves $0,$ that's not $\le C\delta^{\alpha}.$ So did he intend to write inf here instead of sup?

Thanks in advance!

Answer 1

First point: Since $d(\theta, \theta_0)<\delta$ it should be possible to select $\theta = \theta_0$ which gives $\sup=0>-C\cdot0^\alpha$.

Without being specific for this problem, if $x^*=\arg\max a(x)$ then $$ a(x) - a(x^*) \leq 0 $$ Taking inf typically means that an upper bound is easily achievable. The posted requirement (with the modification $d(\theta, \theta_0)>\delta$ ) seems reasonable: $$ \sup_{|x-x^*|>\delta} a(x) - a(x^*) \leq C\delta^\alpha; $$ when $x$ is not identical to $x^*$, there will be a distance between the function values.