1
$\begingroup$

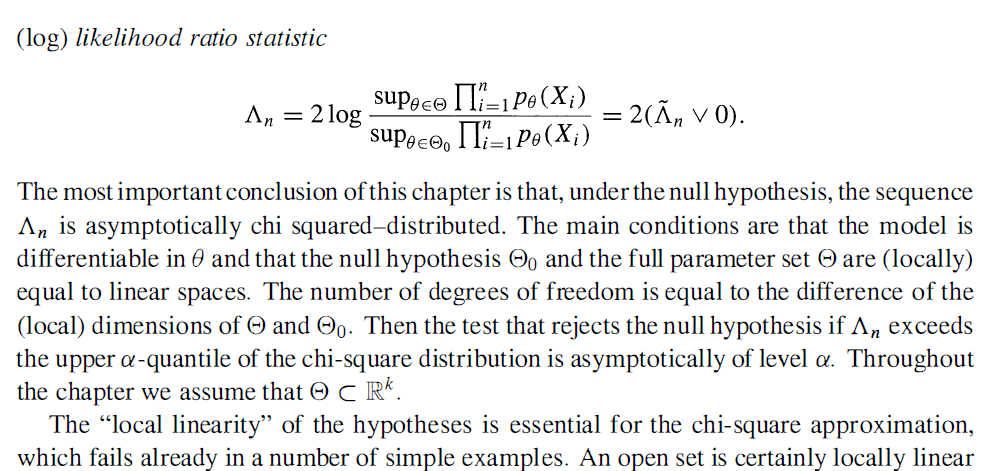
In his book 'Asymptotic Statistics,' Aad van der Vaart when discussing the asymptotic distribution of the log-likelihood-ratio says:

"The most important conclusion of this chapter is that, under the null hypothesis, the sequence Λ (the log likelihood ratio) is asymptotically chi squared-distributed. The main conditions are that the model is differentiable in θ and that the null hypothesis and the full parameter set are (locally) equal to linear spaces."

And then latter says "The local linearity of the hypotheses is essential for the chi-square approximation".

Previous derivations I've seen of this require the usual regularity conditions be satisfied for this property. Regularity conditions make no mention of local linearity, so I'm confused now. Are they equivalent or does local linearity automatically satisfy these regularity conditions?

[enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

In the usual setup, the local linearity assumption comes in even before the usual smoothness assumptions, sometimes explicitly and sometimes just in the notation setup.

In the usual setup, $\Theta$ is (an open subset of) $\mathbb{R}^k$ and $\Theta_0$ is a smooth submanifold of it, and so is locally like $\mathbb{R}^{k-p}$ for some $p$. That's what the book goes on to say, in the sentence you cut off

An open set is certainly locally linear at every of its points, and so is a relatively open subset of an affine subspace.

And the counterexamples are null hypotheses where there's an edge to $\Theta_0$, such as $\theta\leq 0$ in $\mathbb{R}$ or $\|\theta\|\leq 1$. In those cases you don't get $\chi^2$ tests; you get some other more complicated functional of a $k$-variate Gaussian vector as the distribution of the loglikelihood.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thanks for answering this. The point about parameters needing to lie within the interior of the parameter space is usually a requirement explicitly stated by the regularity conditions. Presumably, this idea of local linearity is broader than that. For instance, I interpreted this as meaning that the model needs to be linear with respect to the parameters; that is, the model prediction has a linear relationship with the parameter? $\endgroup$
    – PMTokai
    Commented Feb 14 at 0:25
  • $\begingroup$ No, this has nothing to do with linearity of the model -- differentiability is enough, and that's separate. It really is just saying that the parameter space is locally like ${\mathbb{R}^k}$ rather than being some more complicated shape with an edge or non-flat like the corner of a cube or full of little holes or any of the other horrors that a topologist could think up. $\endgroup$
    – Thomas Lumley
    Commented Feb 14 at 1:54
  • $\begingroup$ Ah, okay. Thank you once again. Hmm, It might be worth getting your perspective on how this problem is described in this paper then. Here, the phrasing suggests that the model needs to be linear, but maybe this is just sloppy wording and the problem stems from the fact that they are really fitting in $\sin(\delta)$ and $cos(\delta)$, and thus the parameter-space does not locally look like $\mathbb{R}^{2}$ since it is constrained by $\sin^2 (\delta) +\cos^2 (\delta) = 1$ ? Paper: arxiv.org/abs/1407.3274 $\endgroup$
    – PMTokai
    Commented Feb 14 at 18:49
  • $\begingroup$ For reference I mean when they say things like: "Similarly, deviations from a $\chi^2$ distribution of the test statistic should be tested for in the search for $\delta$. Indeed, one of the requirements for the validity of the common approach when making sensitivity analyses is that the change in number of events forms a linear space upon variations of $\delta$." $\endgroup$
    – PMTokai
    Commented Feb 14 at 18:56
  • $\begingroup$ and: "This can be understood as follows. One of the requirements for the applicability of Wilks’ theorem is that, when varying the parameter that is being tested for (in this case $\delta$), the subsequent change in the observables used to determine it should constitute a linear space." $\endgroup$
    – PMTokai
    Commented Feb 14 at 18:57

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.