0
$\begingroup$

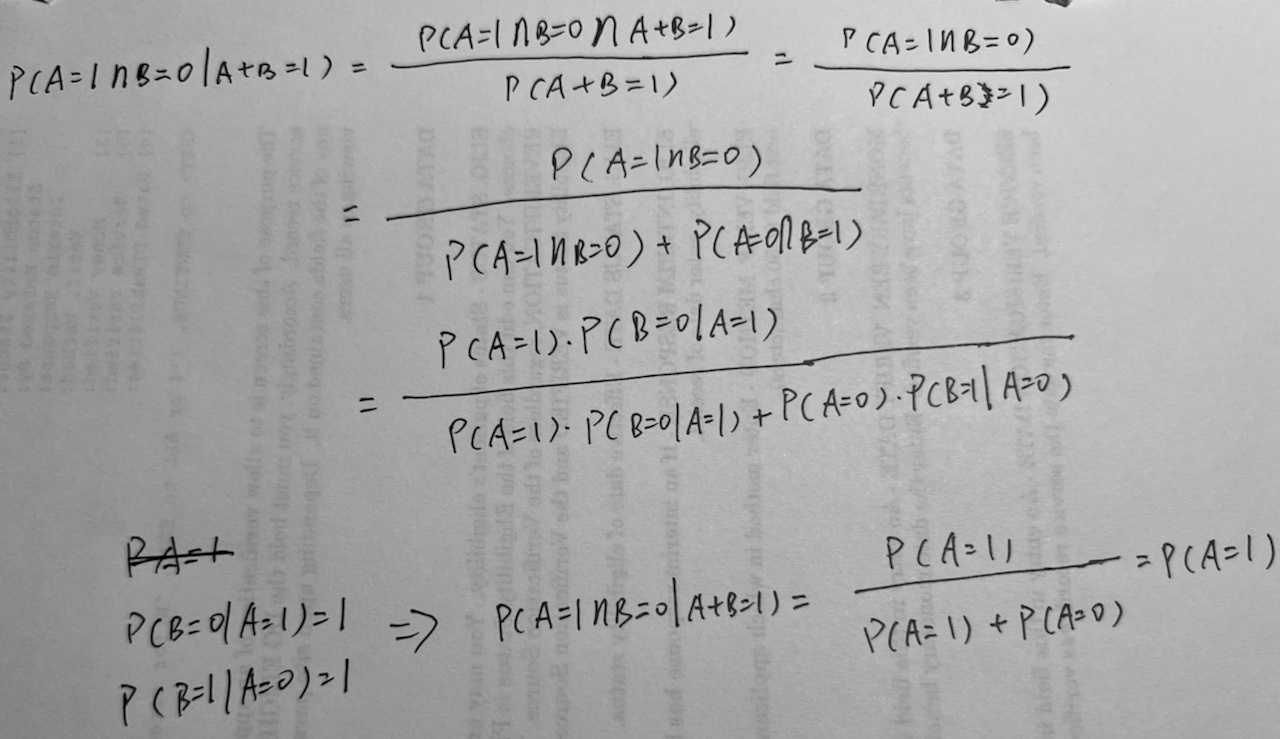
I found the information about the likelihood of conditional logistic regression for paired data is few. The Wiki gives this answer, but I think it is wrong because event Yi1 and Yi2 are dependent, because Yi1 + Yi2 =1. (Yi is a binary random variable). Therefore, how do we solve the conditional likelihood based on the assumption of Yi1 + Yi2 = 1? I have not found any proof yet. Thanks.

enter image description here

Updated on 10May2024

This is my calculation.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

No, Wikipedia is correct. $Y_1$ and $Y_2$ are dependent, but they are dependent only after conditioning on $Y_1+Y_2=1$. You start with $Y_{i1}$ and $Y_{i2}$ independent Bernoulli with $Y_{ij}$ having mean $\mathrm{expit}(\alpha_i+X_{ij}\beta)$ in the population.

At this point you either sample in such a way that $Y_{i1}+Y_{i2}=1$ (matched case-control sampling) or you sample pairs without regard to $Y$ and condition on $Y_{i1}+Y_{i2}=1$ in the analysis (because pairs where that isn't true aren't informative for $\beta$)

Either way, the conditional likelihood for pair $i$ is $P(Y_{i1}=1|Y_{i1}+Y_{i2}=1;\beta)$ or $P(Y_{i1}=0|Y_{i1}+Y_{i2}=1;\beta)$

Improve this answer
$\endgroup$
4
  • $\begingroup$ Hello, Thomas. Thank you for your reply. However, I do not quite understand the proof. I drew a flow of my understanding. $\endgroup$
    – Tom Hsiung
    Commented May 10 at 12:16
  • $\begingroup$ You're still treating $A$ and $B$ as dependent. They are independent Bernoulli prior to conditioning, so that eg $P(A=0|B=1)$ is just $P(A=0)$ $\endgroup$
    – Thomas Lumley
    Commented May 12 at 23:55
  • $\begingroup$ Hi, Thomas. Oh! I got your point. Hmmm, without the conditioning of A + B = 1, there should not have been the correlation between A and B. Thank you very much, pal. $\endgroup$
    – Tom Hsiung
    Commented May 17 at 12:19
  • $\begingroup$ Hi, Thomas. Here I have another question. We know that P(A and B) = P(A | B)*P(B) holds true regardless of the status of the correlation between A and B. When we compute P(A and B), how should we determine whether to treat it as independent between A and B, or to treat them as P(A and B) = P(A | B)*P(B). Thx $\endgroup$
    – Tom Hsiung
    Commented Jun 5 at 12:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.