0
$\begingroup$

In Brockwell and Davis' Introduction to Time Series and Forecasting,

a linear process is defined to be

enter image description here

An ARMA process/model is defined to be

enter image description here

Note that by "stationary", the book means "wide-sense stationary".

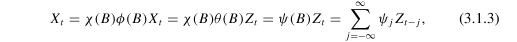
An ARMA process can also be written as a form of linear process enter image description here

  1. Is it correct that a stochastic process is an ARMA process, if and only if it is a linear process and also wide-sense stationary?
  2. Despite that the definition of an ARMA process says $X_t$ only depends on past values of $X$ and $Z$, is it correct that an ARMA process may not be causal, because the solution to (3.1.1) is (3.1.3)? Note a causal ARMA process is defined in (3.1.5) here. Do I misunderstand something?

Thanks and regards!

Improve this question
$\endgroup$
5
  • $\begingroup$ Notice that in a ARMA process, $X_t$ is a function of $Z_t$ and $q$ past $Z_i$ (and $p$ past $X_i$) but no future $Z_i$ (that is, $Z_{t+1}, Z_{t+2}$, etc.) while a linear process has no such restrictions. These kinds of questions are dealt with (both in the stochastic sense here as well as for deterministic sequences) in the signal processing literature, and asking a similar question over on dsp.SE might bring forth useful answers too. $\endgroup$
    – Dilip Sarwate
    Commented Jul 26, 2013 at 12:34
  • $\begingroup$ @DilipSarwate: Thanks! But the definition of ARMA process allows it to depend on future values of $Z_i$. See books.google.com/…. Am I wrong? $\endgroup$
    – Tim
    Commented Jul 26, 2013 at 12:41
  • $\begingroup$ I am looking at the definition (3.1.1) that you have supplied which does not allow $X_t$ to depend on future values of $Z_i$ (or future $X_i$ either for that matter). If you have a different definition in mind, either edit the current question to include it, or ask a different question. $\endgroup$
    – Dilip Sarwate
    Commented Jul 26, 2013 at 12:45
  • $\begingroup$ @DilipSarwate: (1) An ARMA process isn't necessarily causal, because the solution to (3.1.1) is (3.1.3) which shows that an ARMA process may not be causal. (2) A causal ARMA process is defined in (3.1.5) in the link in my last comment, where the definition of an ARMA process is still (3.1.1). Do I misunderstand something? $\endgroup$
    – Tim
    Commented Jul 26, 2013 at 12:56
  • $\begingroup$ @DilipSarwate: I have edited my post to include my confusion in the comments. $\endgroup$
    – Tim
    Commented Jul 26, 2013 at 15:32

1 Answer 1

Reset to default
1
$\begingroup$

An ARMA process corresponds to a rational transfer function. The definition of linear process given is more general, as it allows transfer functions that are not rational.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Can you provide a reference for this claim? $\endgroup$
    – denizen of the north
    Commented Aug 10, 2018 at 17:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.