On the one hand I read in a comment here that:
You can't speak of "eigenvalues" after rotation, even orthogonal rotation. Perhaps you mean sum of squared loadings for a principal component, after rotation. When rotation is oblique, this sum of squares tells nothing about the amount of variance explained, because components aren't orthogonal anymore. So, you shouldn't report any percentage of variance explained.
On the other hand, I sometimes read in books people saying things like:
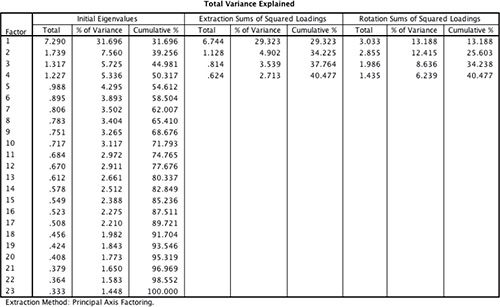
The eigenvalues associated with each factor represent the variance explained by that particular factor; SPSS also displays the eigenvalue in terms of the percentage of variance explained (so factor 1 explains 31.696% of total variance). The first few factors explain relatively large amounts of variance (especially factor 1), whereas subsequent factors explain only small amounts of variance. SPSS then extracts all factors with eigenvalues greater than 1, which leaves us with four factors. The eigenvalues associated with these factors are again displayed (and the percentage of variance explained) in the columns labelled Extraction Sums of Squared Loadings.
That text is from Field (2013), and this diagram accompanies it.
I'm wondering
- Who is correct about whether it's possible to speak of eigenvalues after rotation? Would it matter if it was an oblique or orthogonal rotation?
- Why are the "initial eigenvalues" different from the "extraction sums of squared loadings"? Which is a better measure of total variation explained by the factors (or principal components or whatever method is used)? Should I say that the first four factors explain 50.317% of variation, or 40.477%?
Field, A. (2013). Discovering statistics using IBM SPSS statistics. Sage.