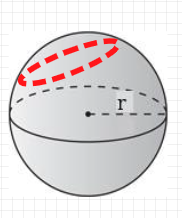
Suppose I have the following scenario:

And I am aware of an algorithm to draw uniformly from (in this case) the 2-sphere. Does this same algorithm readily extend to the situation where I randomly take a "cut/slice" (shown in red above) and what points uniformly over this circle?

Further, suppose now that I have a uniform random sampling algorithm over an arbitrary Riemannian manifold. Would the same principles necessarily apply?