How did Steve Mould predict his child birth time with contraction duration over time?

Question

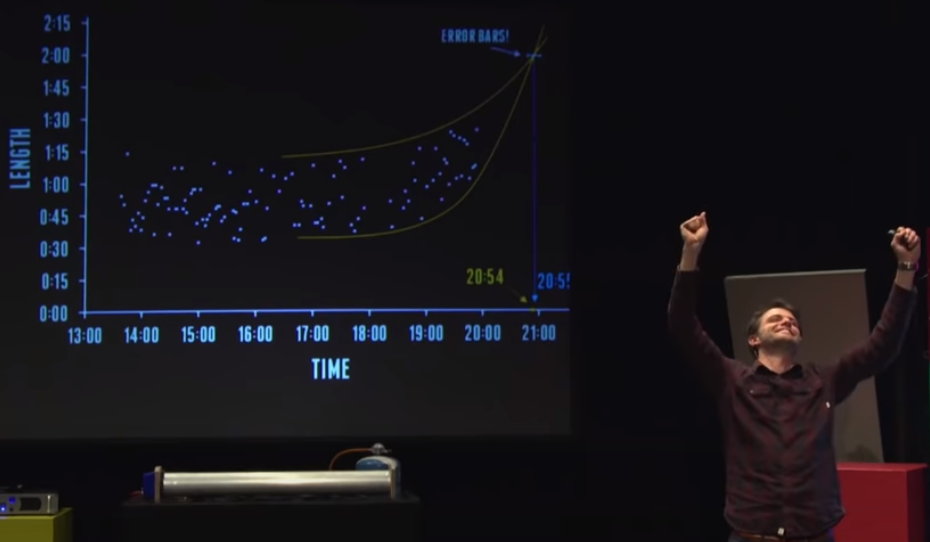
Steve Mould uses contraction data to predict the delivery time of his child [1]. Unfortunately the details from his presentation are sparse:

They are not very predictable. They're quite spread out, but as time goes on it gets more predictable - it's more bunched up there. And there's a wonderful thing you can do with excel. You can track how that changes as a function of time. Is the standard deviation basically. So I was able to create sort of an envelope of possible contraction lengths and then project that into the future. [...] there is point in the future at which my wife's contractions become perfectly regular and that's at 20:55. And it's my working hypothesis that that is the moment my child will be born [2].

Questions:

1. How did Steve Mould calculate the lower and upper bound lines? I cannot figure out how to track how predictable the random variable is becoming. Is it just just confidence interval using the last 10 points?
2. How does Steve extrapolate the lines and predict?
3. How is the intersection of the extrapolated lines calculated?

Answer 1

How did Steve Mould calculate the lower and upper bound lines?

Probably a simple moving-minimum / moving-maximum, which would be easily done in Excel.

How does Steve extrapolate the lines and predict?

Probably just least-squares fitting to a polynomial (looks like it could be a simple quadratic to me).

How is the intersection of the extrapolated lines calculated?

Subtract the two polynomials and find the zero of the difference (specifically, the first root "to the right" of the data points).

and bonus "question" from comments:

I'm perplexed by the hypothesis that the time when there is zero predicted variability in contraction length should correspond to the moment of birth.

Well, obviously it was a guess. It amounts to a hypothesis that the contraction duration is heteroscedastic, and generated by a process where the variance is proportional to the time before birth (or some suitably monotonic function of that time). As a guess, it seems to have worked out pretty well :)