I do not understand why in some figures they put $t_{1-\alpha/2}$. Why is the subscript $1-\alpha/2$ used?
I know that $t_{\alpha/2}$ and $-t_{\alpha/2}$ are used to construct confidence intervals.
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
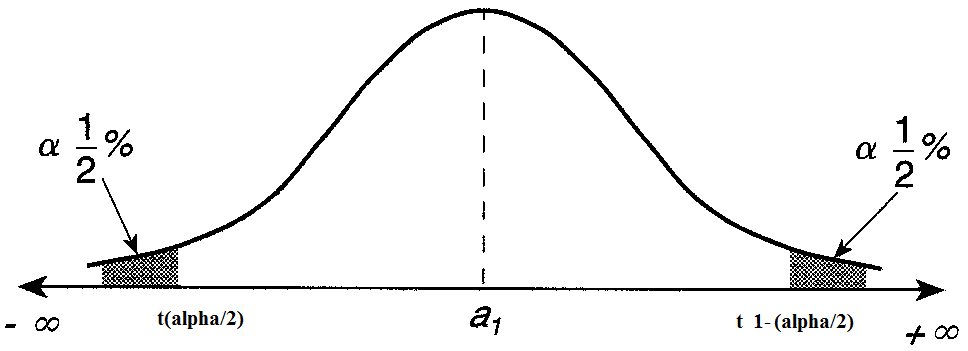
You want a total area in your rejection region of $\alpha$.
If your rejection region is two-tailed, that means there's an area of $\alpha/2$ in each tail.
The upper tail critical value that has and area of $\alpha/2$ above it has an area of $1-\alpha/2$ below it.
The t- critical value that has an area of $1-\alpha/2$ below it is denoted as the $t_{1-\alpha/2}$ value.
For a two-sided confidence interval you want a probability (and hence, area) of $1-\alpha$ between the two bounds, and so $\alpha/2$ in each tails. That is, the same calculations apply.
-
$\begingroup$ so for the first one, -t α/2 and t α/2 , the reasoning is for critical region $\endgroup$– sihouCommented Nov 18, 2015 at 10:10
-
$\begingroup$ while for tα/2 and t1−α/2 is the area of 1−α/2 minus α/2 to have 1 -2*α/2 then to get confidence region 1-α., so both give the same result $\endgroup$– sihouCommented Nov 18, 2015 at 10:12
-
2$\begingroup$ @sihou: $-t_{\alpha/2}=t_{1-\alpha/2}$ because the t distribution's symmetrical. For a skewed distribution you'd have to use an expression like the right-hand one, & that's perhaps a reason for writing it that way even in the symmetrical case. $\endgroup$– Scortchi ♦Commented Nov 18, 2015 at 10:28