I understand that 2 variables may be independent and identically distributed, but I am unable to understand what it means for them to be equal.
I came across a statement of the birthday paradox and was confused by the statement concerning the equality of the random variables mentioned.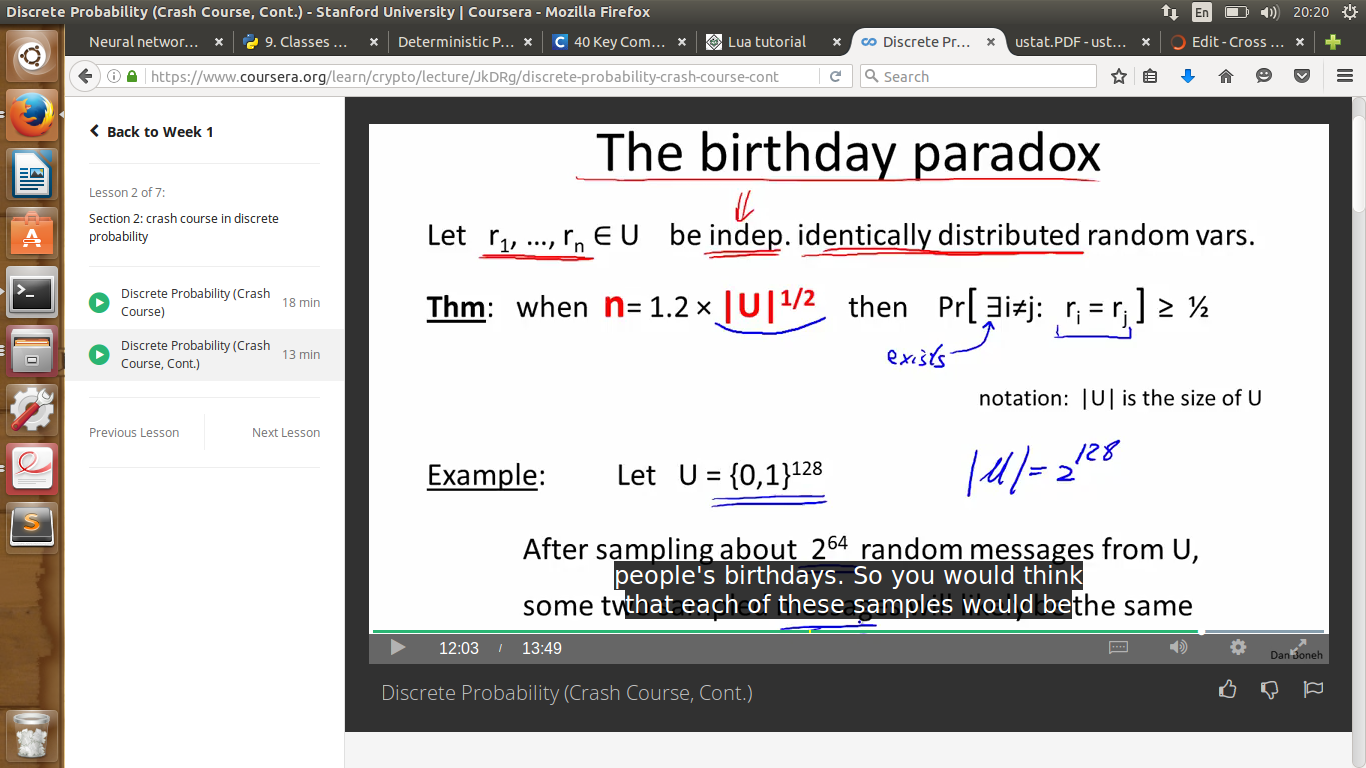 
$\begingroup$
$\endgroup$
4
-
1$\begingroup$ ...and in which context did you find people looking at whether they are equal or not? $\endgroup$– J. M. is not a statisticianCommented Jun 18, 2016 at 12:17
-
$\begingroup$ Can you say where you saw this & provide some of the context? Were they referring to the means being equal, eg? $\endgroup$– gung - Reinstate MonicaCommented Jun 18, 2016 at 12:19
-
$\begingroup$ I have edited the question. I think the image provides sufficient context now. $\endgroup$– Arunabh SaxenaCommented Jun 18, 2016 at 12:23
-
$\begingroup$ The image makes no reference to random variables being "equal." $\endgroup$– whuber ♦Commented Jun 18, 2016 at 14:30
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
What is meant here is not equality of the random variables, it's equality in the value that the random variable took.
In the birthday problem it is assumed every person has a birthday taken with equiprobability from the 365 days of the year (we assume each year has always 365 days). We can say the first person is associated with $X_1$, the second with $X_2$, ... Since all those random variables have the same distribution and are assumed independent they are iid as stated in your video.
Then, what the professor does is saying that the person 1's birthday is one sample drawn from $X_1$ distribution, same for person 2, etc.
Finally, you can say $X_1 = X_2$ to say that person 1 and person 2 had the same birthday. You are here comparing the values taken by the random variables (the samples) but not the random variables themselves.
-
$\begingroup$ So, why do we need to consider n iid variables? Shouldn't we rather say that these are n samples of the same random variable ? $\endgroup$ Commented Jun 18, 2016 at 13:24
-
$\begingroup$ And I still want to know if there is any sense in calling two random variables equal. $\endgroup$ Commented Jun 18, 2016 at 13:24
-
1$\begingroup$ "Equal" means literally that: they are the same mathematical object. See stats.stackexchange.com/questions/50 for various explanations of what a random variable is. $\endgroup$– whuber ♦Commented Jun 18, 2016 at 14:29