(note that this problem is different from this one, since the latter considers primal's Lagrangian)
Hi,
I am trying to figure out the SVM's dual problem. The primal problem is
$${\displaystyle {\text{minimize }}{\frac {1}{n}}\sum _{i=1}^{n}\zeta _{i}+\lambda \|w\|^{2}}\\ {\displaystyle {\text{subject to }}y_{i}(w\cdot x_{i}-b)\geq 1-\zeta _{i}\,{\text{ and }}\,\zeta _{i}\geq 0,\,{\text{for all }}i,} $$
and the dual problem is defined as
$$
{\displaystyle {\text{maximize}}\,\,f(c_{1}\ldots c_{n})=\sum _{i=1}^{n}c_{i}-{\frac {1}{2}}\sum _{i=1}^{n}\sum _{j=1}^{n}y_{i}c_{i}(x_{i}\cdot x_{j})y_{j}c_{j},}\\
{\displaystyle {\text{subject to }}\sum _{i=1}^{n}c_{i}y_{i}=0,\,{\text{and }}0\leq c_{i}\leq {\frac {1}{2n\lambda }}\;{\text{for all }}i.}
$$
I understand how the hyperplane can be derived, and according to Wikipedia
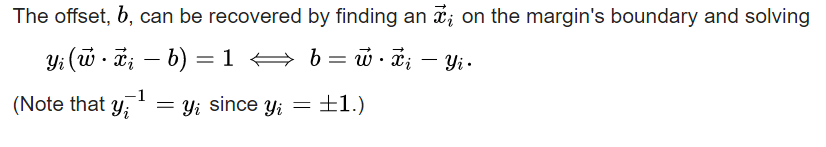
However, I find it difficult to understand how to find $b$ in practice. How can I find an instance on the margin's boundary?
Can somebody provide an explanation for finding the offset?
