1
$\begingroup$

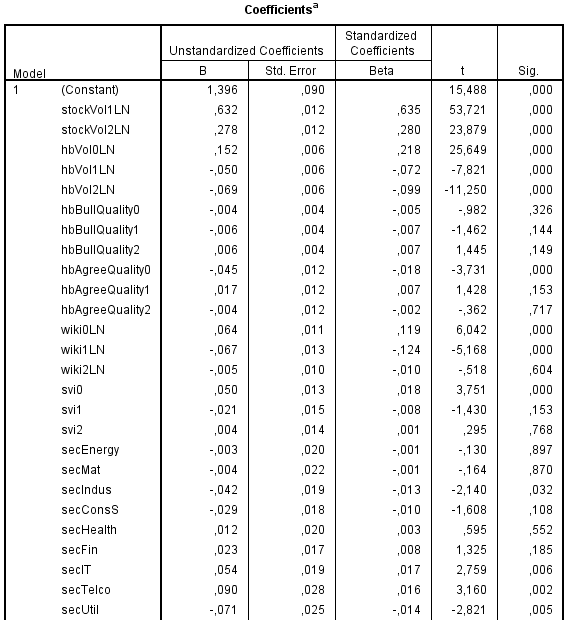
Consider the following (partial) regression results, in which the explainatory value of 5 independent variables are measured for 3 dependent variables. In this post, I'm referring to the models (1d), (2d) and (3d). For each model, the first column is the coefficient, the second the p-value:

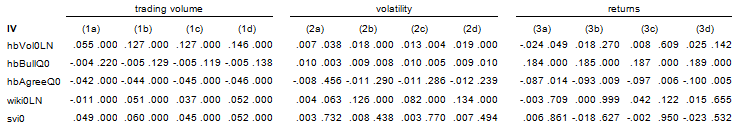
enter image description here

In my thesis, I defined several hypotheses, a.o.

  1. if hvVol0LN increases, trading volume increases
  2. if hbVol0LN increases, returns increase
  3. if hbAgreeQ0 decreases, returns increase
  4. if svi0 increases, trading volume increases

It is now time to check whether these assumptions were right. I just want to double check whether I'm interpreting these results right:

  1. hypothesis accepted: there is a relatively strong (r=.146) and statistically significant (p=.000) relationship
  2. hypothesis rejected; the relationship is very small to begin with, but what is more it is statistically insignificant (p=.142). It would only be significant at the 14,2% significance level
  3. hypothesis accepted at 5% level: the relationship between hbAgreeQ0 and returns is indeed negative and relatively strong (r=-.100), and statistically significant at the 5% level (p=.005). If p would have been larger than .005, the relationship would not be statistically significant, since in practive significance levels of 1% to 5% are accepted (depending on the type of research, of course)
  4. hypothesis accepted: the relationship is significant (p=.000) but not very strong ('r=.052')

Is this a correct way of describing the outcome of the hpyotheses? In other words:

  • can you derive the acceptance / rejection directly from the regression output, and
  • am I interpreting the r and p-values correctly?

Your help in finally finishing my thesis is greatly appreciated :-)

edit: some SPSS regression output to be more clear of what I mean by r

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

People often speak of rejecting the null hypothesis, rather than accepting the alternative, but you seem to have that part right.

Are you confusing 'r' (the correlation) with the parameter estimate from a regression? They are not the same thing. Coefficients are usually called $b_p$ where p ranges from 1 to the number of IVs in your regression. You call them 'coefficient' in the first part of your message.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for your quick reply. I have posted regression analysis from SPSS (see OP, dependent variable is trading volume). Assume the hypothesis is " if hbVol0LN increases, trading volume increases", then I would say: accepted (r=.152, p=.000, see columns B and Sig.). When reporting a regression equation from this output, I would say trading volume = 1,396 + 0.152 * hbVol0LN - .050 * hbVol1LN + ...). Am I doing this wrong? Or do you mean that r is used when taling about correlations, but when taling repgression I should say "accepted, because b(p)=.152, p=.000"? $\endgroup$
    – Pr0no
    Commented Sep 1, 2012 at 21:39
  • 1
    $\begingroup$ You are doing it right, but I would say, rather, "The null hypothesis is rejected" and, yes, I'd use b instead of r. Oh, and I'd say p < .001 instead of p = .000. That's a quirk of SPSS output; but p is never exactly 0. $\endgroup$
    – Peter Flom
    Commented Sep 1, 2012 at 21:45
  • $\begingroup$ that's great. So a correlation coefficient (from the correlation table) is abbreviated to r ("there is a big correlation between A and B because r=.840, p<.001") and regression parameter estimate to b ("X explains Y because b=.158, p<.001")? As a final question, please explain your comment "rejecting the null hpyothesis". My hypotheses are listed in the OP. The 1st H(0) is accepted, the 2nd H(0) is rejected, the 3rd and 4th H(0)'s are accepted. I don't believe I am, as you say, "accepting the alternative"? $\endgroup$
    – Pr0no
    Commented Sep 1, 2012 at 22:04

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.