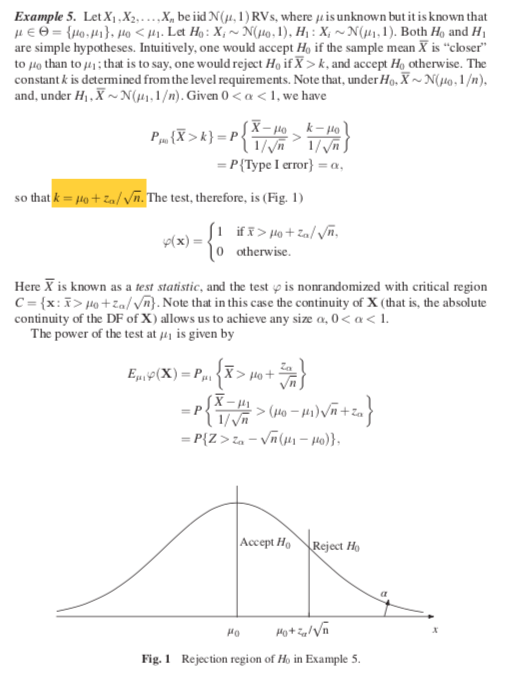
In the following example, can someone help understand how the value for $k$ (highlighted in yellow) is derived below? How is $z_\alpha$ introduced in there?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
We want to find the value $k$ such that it is exceeded by the sample mean with probability $\alpha$ if the null is true.
In the display, the second line subtracts $\mu_0$ and divides by $1/\sqrt{n}$ to get a standard normal r.v. under the null. By definition, a r.v. exceeds its $1-\alpha$-quantile with probability $\alpha$. $z_\alpha$ is this quantile. Hence, $$ \frac{k-\mu_0}{1/\sqrt{n}}=z_\alpha $$ Now, solve for $k$.
answered Dec 17, 2018 at 9:51
-
$\begingroup$ Thanks for the explanation. Can you please give me a reference where I can read more about quantiles and the fact that random variable exceeds its $1-\alpha$-quantile with probability $\alpha$ and that we denote this quantile by $z_\alpha$? Much appreciated! $\endgroup$– cbroCommented Dec 17, 2018 at 11:49
-
$\begingroup$ en.wikipedia.org/wiki/Quantile has further discussion. $z_\alpha$ is just notation. $\endgroup$ Commented Dec 17, 2018 at 12:48