Best measure of central of tendency

Question

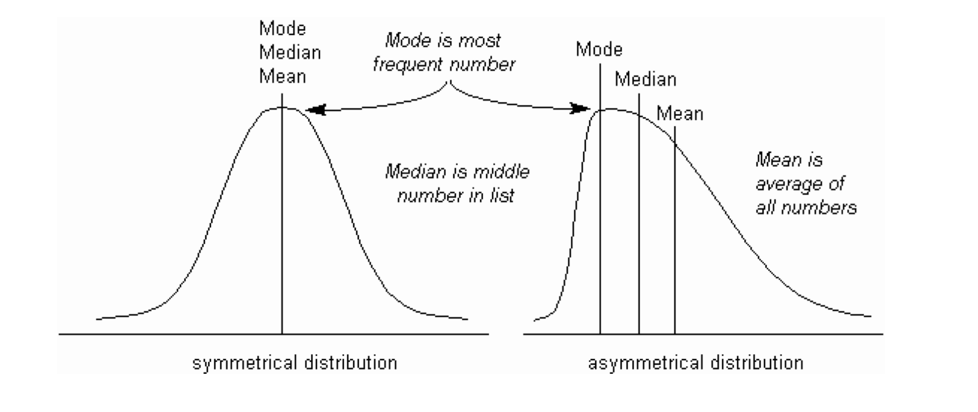
I just started learning stats a few weeks ago well my question is that as we know that the mean, median, and mode is the central tendency of the data and its suggested that we shouldn't go with only one of these measures since few circumstances can affect them well what we should consider the central tendency of the data if their mean, median and mode are telling completely different story about the data

example

mean = 43.26
median = 14
and
mode = 9

and my question is what should we interpret from these measures what would be the better estimate for the central tendency

Answer 1

Different situations call for different answers. Applied statisticians should find the measure that answers the underlying question best.

Consider the following sentence:

Most people have above average number of legs

Most people have 2 legs, some have only one or none. So the mean is probably 1.9...

If someone in the street asked you "How many legs do people have?" they will usually expect the answer "two legs", which is the mode. The mode is often "the normal thing". If, however, you were in a position where you would have to plan a stock of lower limb prosthesis for a country far away, you would want to multiply the mean with the population size. In many cases where you would like to assess a mean from a small sample but are afraid of outliers, the median will be a better estimator.

So the question for the best measure is not a universal mathematical question nor does is necessarily depend on what you measure, but it depends on whatever real world problem you try to tackle.

Answer 2

In my opinion, the answer should be dependent on your distribution shape. E.g., if you have a bell-shaped density, you may consider using mean as an informative estimator. If you have a bit of outliers or you have a skewed distribution or you distribution does not have a well defined mean - you may use median. If you have multi-modal distribution, you may use mode.

All these estimators are essentially different and provide different information about your underlying random variable.

Another thing worth to be discussed (except the deep underlying differences in what these estimators mean) is the efficiency of the estimation and the breakdown point. Mean is the most efficient estimator (your estimation will be as close to the true value using the sample of size that you have). Median is much more robust (has almost 50% breakdown point), but much less effective. Lehman-Hodges estimator is somewhere in between. The mode, which is often obtained via Kernel Density Estimation, is not efficient at all and it makes sense to use it only if you have >50% of "outliers" - even in this case you need to be very careful with the kernel that you use, e.g., the default kernel in R is kept there for historical reasons and should not be used.

These are my opinion and may be wrong.

Image credit: https://www.tutor2u.net/geography/reference/mean-median-and-mode