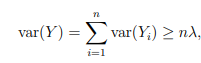0
$\begingroup$

For $Y_i$ independent $ \sim \mathcal{Poisson}(λ_i)$, $i = 1, \dotsc,n$, I want to assume $λ_i$ has mean $λ$ and variance $σ^2$. With this I want to show the following, but I am not sure how to show it. All of the websites I've checked, it's simply just been "this is the case", but it doesn't give an explanation why or how and I would like to understand.

enter image description here

If you have any links to websites that may help, that would be greatly appreciated.

Improve this question
$\endgroup$
2
  • $\begingroup$ Lots of similar questions with answers. Must be a dup in there ... $\endgroup$
    – kjetil b halvorsen ♦
    Commented Feb 26, 2020 at 12:39
  • 1
    $\begingroup$ @kjetilbhalvorsen I looked through and did not find a duplicate to my question. Most have to do with finding overdispersion in R, which is not what I am trying to do. $\endgroup$
    – Fire
    Commented Feb 26, 2020 at 16:07

1 Answer 1

Reset to default
0
$\begingroup$

I will only give a hint, the law of total variance is your friend. Start out with $$ \DeclareMathOperator{\E}{\mathbb{E}} \DeclareMathOperator{\V}{\mathbb{V}} \V Y = \V\left( \sum_i Y_i\right) =\\ \E \V \sum_i Y_i \mid \lambda_i + \V \E \sum_i Y_i \mid \lambda_i = \dotsm $$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.