Actually, it's relatively simple to obtain formulas for the entire distribution as well as an easy procedure to compute any moment of it.
For $n=1,2,3,\ldots,$ let $f_n(p) = \Pr(X=n)$ with $p=0.6.$ Define
$$F_p(t) = f_1(p)t + f_2(p)t^2 + \cdots + f_n(p)t^n + \cdots$$
(the probability generating function). The problem asserts
$$F_p(t) = p\,t + (1-p)F_p^2(t),$$
a quadratic equation with solutions
$$F_p(t) = \frac{1}{2(1-p)}\left(1 \pm \sqrt{1 - 4p(1-p)t}\right).$$
Only the solution with a minus sign makes sense (because the other yields a negative value for $f_2(p)$). Expanding it as a formal power series in $t$ (using, for instance, the Binomial Theorem) gives
$$F_p(t) = \frac{1}{2(1-p)} \sum_{n=1}^\infty (-1)^{n-1} \binom{1/2}{n} \left(4p(1-p)\right)^n\,t^n = \sum_{n=1}^\infty f_n(p)\,t^n,$$
from which we can read off the entire distribution of $X$ term by term. Here's a plot of the log probabilities up to $n=80$ created using this formula:
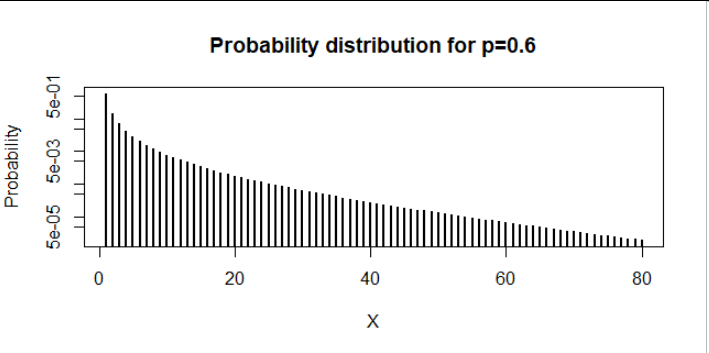
(In R:
f <- function(p=0.6, n=1:80) (-1)^(n-1) * choose(1/2, n) * (4*p*(1-p))^n / (2*(1-p))
plot(f(), type="h", log="y")
)
Moreover,
$$E[X] = \frac{\mathrm{d}}{\mathrm{d}t}F_p(t)\bigg|_{t=1} = \frac{p}{\sqrt{1-4p(1-p)}} = 3$$
and
$$E[X(X-1)] = \frac{\mathrm{d}^2}{\mathrm{d}t^2}F_p(t)\bigg|_{t=1} = \frac{2p^2(1-p)}{\sqrt{\left(1 - 4p(1-p)\right)^3}} = 36,$$
whence
$$\operatorname{Var}(X) = E[X(X-1)] + E[X] - E[X]^2 = 36 + 3 - 3^2 = 30.$$

