Why are Poisson distribution and Exponential distribution special case of Gamma distribution?

Question

I am aware that Gamma distribution is used as a conjugate prior distribution for various types of rate parameters such as in Poisson distribution and Exponential distribution.

People say that Exponential distribution is a special cases of the gamma distribution. How to understand is sentence? Can anyone share some comments on this?

Answer 1

The Gamma and the exponential distributions have several different parametrizations. Let's use:

• Gamma: $f(x)=\frac{\beta^{\alpha}}{\Gamma(\alpha)}x^{\alpha-1}e^{-\beta x}$, where:
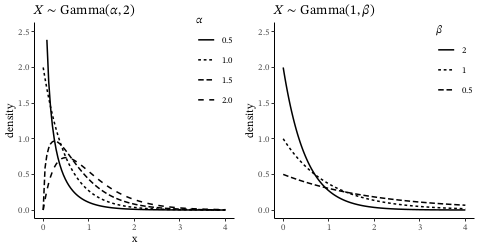
  • $\alpha$ is a shape parameter: if $0<\alpha\le 1$ then $f(x)$ is strictly decreasing, if $\alpha>1$ then it is bell-shaped;
  • $\beta>0$ is a rate (or inverse scale) parameter, the rate at which $f(x)$ increases or decreases;
  • $\Gamma$ is the gamma function.

• Exponential: $f(x)=\lambda e^{-\lambda x}$, where
  • $\lambda>0$ is a rate (or inverse scale) parameter, the rate at which $f(x)$ decreases.

Since an exponential density is strictly decreasing, a Gamma density can have similar shape only if $\alpha\le 1$.

If you try $\alpha=1$ you get: $$f(x)=\frac{\beta^{\alpha}}{\Gamma(\alpha)}x^{\alpha-1}e^{-\beta x}=\frac{\beta^1}{\Gamma(1)}x^0e^{-\beta x}=\beta e^{-\beta x}\qquad (\Gamma(1)=0!=1)$$ which is the density of an exponential random variable.

The exponential distribution is the probability distribution of the time (a continuous variable) between events in a Poisson point process, but a Poisson distribution is not a special case of a Gamma distribution (see Xi'an's comment).

Answer 2

There are various ways to show two probability distributions are equal, examples include showing equality for any of the following:

• Probability functions (pmf or pdf)
• Cumulative distribution functions
• Moment generating functions
• Characteristic functions

For the gamma distribution there are various parameterizations, as shown on its Wikipedia page. We know the exponential only has one parameter, while the gamma has two, so we want to transform away one of these parameters. The pdf of the gamma distribution here, https://en.wikipedia.org/wiki/Gamma_distribution, with alpha and beta is already fairly similar to the exponential and substituting $\alpha = 1$ and noting that they have the same support gives the answer.