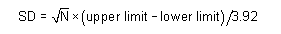0
$\begingroup$

I am planning a clinical study and need to determine the needed sample size. My primary endpoint will be measured in blood pressure (mmHg). Many studies already exist for this, but they do not often provide sdev for the measurements of blood pressure in hypertensive patients. I did, find a study in Germany (DEGS) that provided the mean blood pressure for treated hypertension and I based my sample size estimation on this. Specifically, I used the values from Treated hypertension in Table 3 Total, namely 130.3 mmHg (129.2 - 131.4) with a n of 7095*0.154 (see DEGS Table 2 Total lower row)

Following the approach described here, I then used

getting SD from 95% CI and means

to arrive at a sdev of 18.55125 mmHg.

My question is now: did I do this right? Thank you all for your guidance!

Improve this question
$\endgroup$
7
  • $\begingroup$ How did you get $3.92$? $\endgroup$
    – Dave
    Commented Oct 5, 2020 at 11:59
  • $\begingroup$ The Cochrane statistics guide I used gave 3.92 as the appropriate measure since it is 2*(standard error) = 2*1.96. It's the link I labelled as "here" $\endgroup$
    – P.Weyh
    Commented Oct 5, 2020 at 12:38
  • 1
    $\begingroup$ I have no idea where you got 7095 * 0.154 as the sample size from as I do not see it in Table 2 of the reference but your approach seems sound. As a sanity check ask yourself whether you would expect most BP to be within 37 either way of the mean. $\endgroup$
    – mdewey
    Commented Oct 5, 2020 at 13:11
  • $\begingroup$ Do you know why they use 2*1.96? $\endgroup$
    – Dave
    Commented Oct 5, 2020 at 13:34
  • $\begingroup$ @Dave I am really not sure, I had been hoping to get some insight into that on this platform :) $\endgroup$
    – P.Weyh
    Commented Oct 5, 2020 at 14:20

1 Answer 1

Reset to default
1
$\begingroup$

The usual approach is to reverse the computation for the confidence interval so we take the half width of the confidence interval and then divide it by the relevant quantile of the normal or Student's $t$ distribution to give the standard error. Multiplying this by the square root of the sample size yields the standard deviation.

The only reservation about this is that Brown in a paper entitled On the use of a pilot sample for sample size determination suggests that if the standard deviation comes from a small pilot study it might be better to use instead the upper 80% confidence limit. This is because the distribution of the standard deviation is skewed and so the obtained value will under-estimate the true value. If the study being used for the estimate is large this is unlikely to make much difference.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you for the response! Would that then equate SD=sqrt(N)*(upper limit - lower limit)*0.5/1.96 ? I am not sure what you mean by the "relevant quantile of the Students t distribution"? $\endgroup$
    – P.Weyh
    Commented Oct 6, 2020 at 7:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.