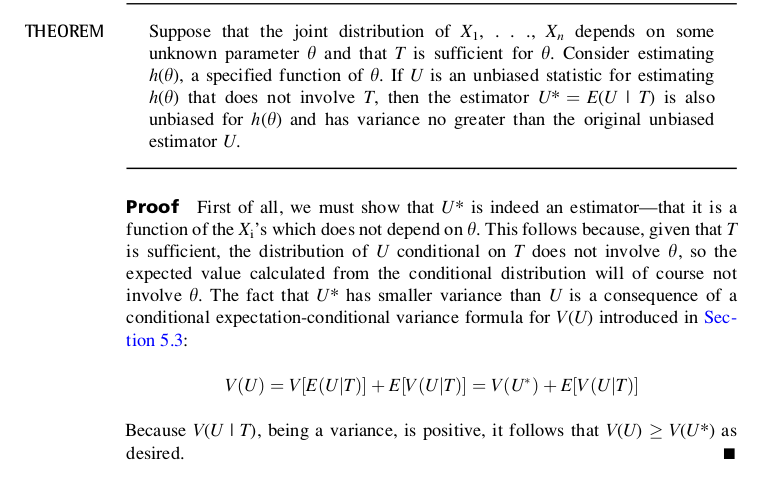
This is an excerpt from "Modern mathematical statistics with applications" by Devore et al. What puzzles me is that the estimator cannot help being dependent on $\theta$, since the sample depends on the parameter.
$\begingroup$
$\endgroup$
0
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
5
You are right that any sensible estimator will be a (non-constant) function of the data (except in some special, arguably pathological, cases, such as my example here). So, it is correct to say that a reasonable estimator does depend on $\theta$ through its dependence on the data. But, I'm pretty sure all that is meant by the sentence
Show that $U^{\star}$ is indeed an estimator - that it is a function of the $X_i$'s that does not depend on $\theta$
is that the formula for an estimator cannot contain the parameter. This is to exclude things like $\hat{\theta} = \theta$, which would be a perfect estimator (even if you had no data!!) but you'd need to be psychic in order to calculate it :-)
As noted in the passage you pasted, since $T$ is a sufficient statistic, the distribution of any statistic, e.g. $U$, conditional on $T$, will not depend on $\theta$. Therefore, $U^{\star} = E(U|T)$ cannot depend on $\theta$, ensuring that it will have the property in question.
-
2$\begingroup$ +1 This question uncovers an interesting ambiguity in the language of this (well-received, popular) textbook: "depend on $\theta$" could mean at least three distinct things! (1) $\theta$ does not explicitly appear in the formula. (2) Although $\theta$ might appear in the formula, the formula is invariant under changes to $\theta$. (3) $\theta$ is viewed as a (perhaps constant) random variable and "depend" could be intended in the sense of dependence of random variables. Unfortunately, the attempted clarification ("the distribution ... does not involve $\theta$") is too vague to help much. $\endgroup$– whuber ♦Commented Mar 18, 2013 at 16:57
-
$\begingroup$ Hi @whuber - I'm not quite sure what you mean with (2). I'm trying to think of an estimator that has that property. Do you mean that the way you calculate the estimator would be the same regardless of $\theta$? That seems to be equivalent to $\theta$ not appearing in the formula. Otherwise, you'd again need to be psychic to calculate the estimator, right? If you meant invariant in the sense that the numerical value of the estimator remains the same regardless of the value $\theta$ then that doesn't sound like a very good estimator :-) Can you clarify? $\endgroup$– MacroCommented Mar 18, 2013 at 18:08
-
1$\begingroup$ It's a subtle difference, but it's real. As a trivial example, after observing $k$ successes in $n$ iid Binomial trials with parameter $\theta$, obviously $\theta$ appears in the (admissible) estimator "$(k+1)/(n+\log(\exp(\theta)^2)/\theta)$," but it nevertheless is valid because it does not vary with $\theta$. More subtly (and still trivially) in an iid Normal sampling problem, the estimator $\hat{\mu}=\bar{x}+1000\mathbb{I}_{\bar{x}\in\mathbb{Q}}$ not only involves $\theta$ but actually varies with it--yet the chance that it is not constant is zero and $\hat{\mu}$ is as good as they come. $\endgroup$– whuber ♦Commented Mar 18, 2013 at 18:10
-
$\begingroup$ I guess I'm still missing your point. In the first estimator, $\log( \exp(\theta)^2 ) = 2 \theta$, so $\theta$ actually cancels out of the expression and it seems better to just write it as $(k+1)/(n+2)$. I think I'm really missing your point with the second one. I don't see a $\mu$ in there and it seems that $P( \overline{x} \in \mathbb{Q}) = 0$ since the probability of $\overline{x}$ being an integer is zero. So, $\hat{\mu} = \overline{x}$ with probability $1$, which doesn't involve $\theta$. I'm probably being dense. If it's too long for a comment, maybe we can do this in chat sometime. $\endgroup$– MacroCommented Mar 18, 2013 at 23:05
-
$\begingroup$ Sorry about the typo: that second estimator should have been $\hat{\mu}=\bar{x}+1000\mu\mathbb{I}_{\bar{x}\in\mathbb{Q}}$. The distinction in the first case is between a formula and its values. (BTW, your equation of $\log(\exp(\theta)^2)/\theta$ with $2$ is not fully correct because it fails for $\theta=0$, where my formula is undefined.) $\endgroup$– whuber ♦Commented Mar 18, 2013 at 23:33