1
$\begingroup$

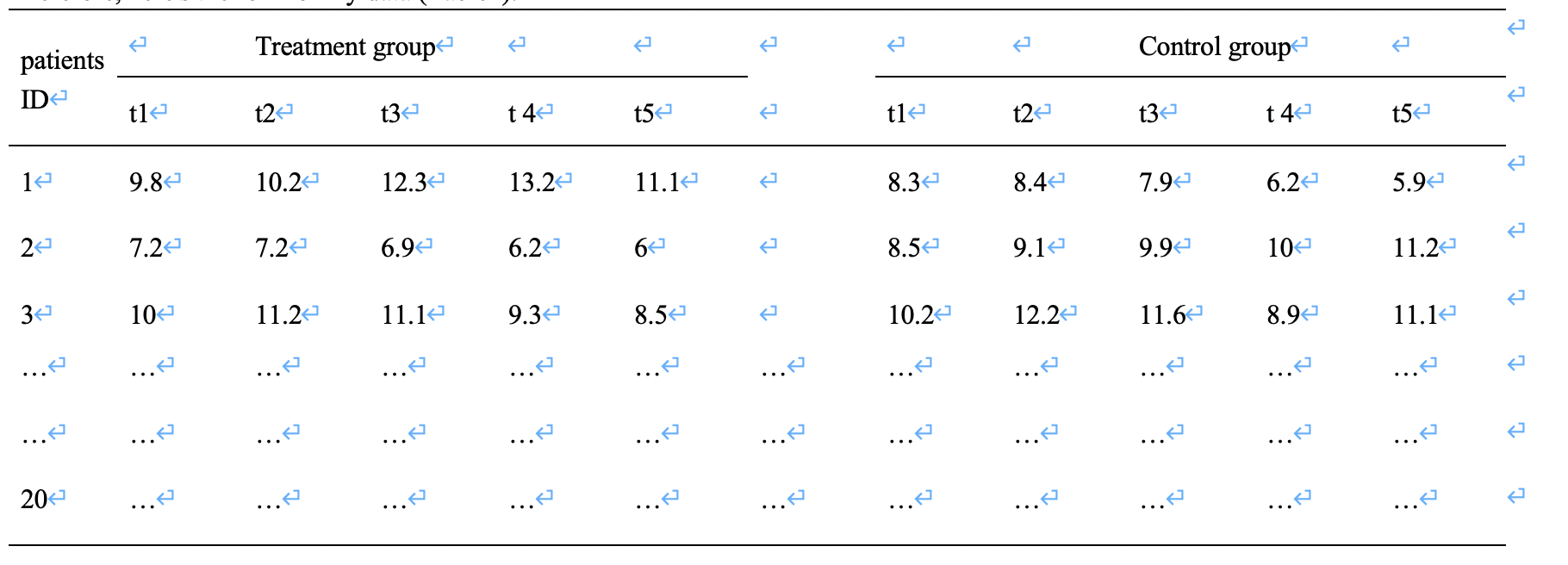
I am doing a study to evaluate the effect of treatment on eyes. The purpose was to explore whether the level of X protein is different between treatment group (sick eye) and normal control group (normal eye).

Twenty patients were selected in our study and accepted the treatment for the sick eye.

After the treatment, for each patient, we tested the level of X protein in the treatment group (sick eye) and normal control group (normal eye) at 5 time point, respectively (1 month, 3 month, 6month, 9 month, 12 month).

Therefore, here's the form of my data (attached figure).

How to describe the design in the present study?

1、Two repeated measured factors design?

We think the time factor is a repeated measured factor, and the treatment factor is the other repeated measured factor. However, could the treatment factor be considered as a repeated measured factor? Because it only has two levels (Treatment group vs. control group).

2、The sick eye is selected in treatment group, the normal eye is control group.

Did it is a matched pairs design? And it is a matched pairs combine two repeated measured factor design?

So, which design it is?

How to conduct the analysis? Linear Mixed model or GLMM or Multilevel model？

Thanks a lot!

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

The problem with trying to come up with a precise, technical name for your design is that not everyone understands it, or agrees with the name, so it might not help (see, for example 'mixed anova', 'structural equation' or 'hierarchical regression').

In your design, how will you treat time? Is that categorical or continuous?

"Two repeated measured factors design" is not wrong, but you probably want to add some more description, because that doesn't provide enough information. There are many ways to analyze it - I'd use a linear mixed model (probably).

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thank you very much for your solution and your suggestion！ In the study, the time was treated as a categorical variable. Does the study include the matched pairs design or not? " the sick eye is selected in treatment group, the normal eye is control group. " Should we consider it (treatment factor）as a self-matching factor ？ If it is, how to manipulation the self-matching factor in repeated measured analysis? : 1. only consider the self-matching factor（treatment factor）as fixed factor in LMM ? 2. Should we analysis it by special method? Thanks! @Jeremy Miles $\endgroup$
    – skywalker21th
    Commented Sep 8, 2021 at 1:32
  • $\begingroup$ I would call eye status (sick or not) a predictor in the model. $\endgroup$
    – Jeremy Miles
    Commented Sep 8, 2021 at 5:28
  • $\begingroup$ Thanks! Actually, It's not similar with Paired-Samples T Test . The matched pairs factor is not considered as a special factor when we conduct a repeated measured design ? $\endgroup$
    – skywalker21th
    Commented Sep 10, 2021 at 7:21
  • $\begingroup$ Not sure what you're asking. What's a special factor? $\endgroup$
    – Jeremy Miles
    Commented Sep 10, 2021 at 16:54
  • $\begingroup$ Forgive me the imprecise description. But, I always confused that if a factor was considered as the matched pairs factor in the design, whether we should treat it as the the matched pairs when we conduct the statistic analysis. The question is: Is there a statistic method for special treating self-matching factor similar with the paired t test (sorry, the statement may not appropriate ) when we analysis the repeated measured data by LMM method or GLMM or GEE ? Thanks a lot！ @Jeremy Miles $\endgroup$
    – skywalker21th
    Commented Sep 13, 2021 at 4:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.