3
$\begingroup$

If $(X,Y)$ and $(X+Y,Z)$ both follow nondegenerate bivariate Gaussian distributions, is it possible that $(X,Y,Z)$ follow a nondegenerate trivariate distribution that is not Gaussian?

I want to make a statement about adding random variables, e.g. $R_1,R_2,R_3$, using Gaussian copulas:

  1. Add $R_1$ and $R_2$ using a prescribed bivariate Gaussian copula.
  2. Add $R_3$ and $R_1+R_2$ using another prescribed bivariate Gaussian copula.

This is equivalent to adding $R_1$, $R_2$, $R_3$ using some trivariate Gaussian copula. Is the statement true? I am leaning towards not, and I think the way to create a counter example would be similar to Is joint normality a necessary condition for the sum of normal random variables to be normal?? But it seems really hard.

Improve this question
$\endgroup$
4
  • $\begingroup$ Let $X=-Y$ and suppose $(X,Z)$ is not bivariate Gaussian but $Z$ is Gaussian. $\endgroup$
    – whuber ♦
    Commented Oct 4, 2021 at 11:18
  • $\begingroup$ @whuber But that would lead $(X+Y,Z)$ not to be a bivariate Gaussian $\endgroup$
    – user2961927
    Commented Oct 4, 2021 at 20:39
  • $\begingroup$ Not so: it is indeed a bivariate Gaussian. It just happens to be a degenerate one. Proof: when $Z$ has mean $\mu$ and variance $\sigma^2,$ its characteristic function is $$E[\exp(it(X+Y)+isZ]=\exp(i\mu s - \sigma^2 s^2/2).$$ Because its logarithm is a linear function of $\mu$ and a quadratic function of $\sigma,$ this is a Gaussian distribution by definition. $\endgroup$
    – whuber ♦
    Commented Oct 5, 2021 at 14:01
  • $\begingroup$ @whuber Interesting.. in my mind I've always excluded collapsed distribution from the category of being multivariate. I should change the description. Thanks $\endgroup$
    – user2961927
    Commented Oct 5, 2021 at 16:39

1 Answer 1

Reset to default
3
$\begingroup$

Let $Z_i,$ $i=1,2,3,$ be independent standard Normal variables. Define

$$\begin{aligned} X &= Z_1 + Z_2\\ Y &= Z_1 - Z_2\\ Z &= Z_3\operatorname{sgn}(Z_2Z_3). \end{aligned}$$

Because $(X,Y)$ is a linear combination of the bivariate Normal variable $(Z_1,Z_2)$ it, too, is bivariate Normal and $X+Y=2Z_1$ has a Normal distribution.

$(2Z_1, Z_3)$ also has a bivariate Normal distribution. Multiplying its second component by $\operatorname{sgn}(Z_2Z_3)$ does not change that distribution (because it merely randomly changes the sign of $Z_3$ and the symmetry of $(2Z_1, Z_3)$ implies changing the sign of either component does not alter the distribution). Consequently $(X+Y, Z)$ has a bivariate Normal distribution.

However, $(X,Y,Z)$ is not trivariate Normal. This becomes obvious when you realize it is the sum of $(Z_2, Z_3\operatorname{sgn}(Z_2Z_3))$ and the independently distributed Normal variable $(Z_1, 0).$ The former is clearly non-Normal--all its probability is concentrated in the first and third quadrants--and adding a Normal variable merely "smears out" this problematic behavior without curing it.

Here is a scatterplot matrix of 2,000 randomly generated draws to illustrate.

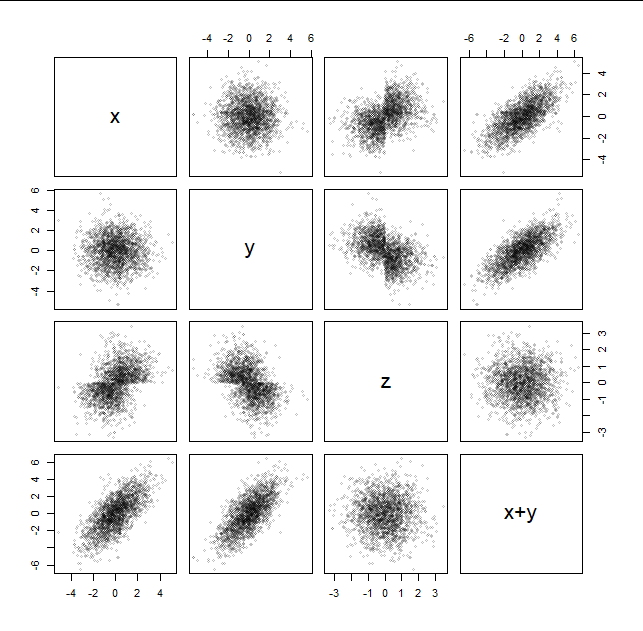
Figure

The question requires that the $X-Y$ plot be Normal as well as the $(X+Y)-Z$ plot. The obvious non-Normality of the $X-Z$ and $Y-Z$ plots demonstrates this is a counterexample.

This is a nice example of why it's rarely effective to limit exceptional behavior of probability distributions by barring "edge cases" such as degenerate versions of families of continuous distributions, provided the problematic behavior can survive even a tiny perturbation of the distribution.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.