1
$\begingroup$

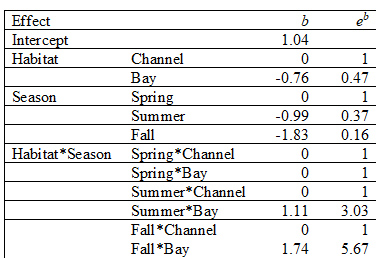
Trying to understand count distributions of a fish species over two habitat types (class variable) and three seasons (class variable). The table below shows the results of a hurdle negative binomial model. I am only showing the count portion of the model. I exponentiated the slopes and can see that counts in bays are 0.47 of those in channel, and counts in summer are 0.37 of those in spring. I am not sure how to integrate the interaction into these estimates. In other words, how does the 0.47 value for bays changes in, say, summer. Thanks in advance.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

I exponentiated the slopes and can see that counts in bays are 0.47 of those in channel, and counts in summer are 0.37 of those in spring.

With your interaction terms, those calculations only hold at reference conditions of the other predictors. "Counts in bays are 0.47 of those in channel" only in Spring; "counts in summer are 0.37 of those in spring" only in Channel.

As your analysis seems to use standard treatment coding, the $b$ coefficients for Summer and Fall represent differences from Spring in Channel. The Summer*Bay and Fall*Bay interaction coefficients are the additional differences beyond what you would get for the corresponding Channel predictions.

The best way to proceed is to add up all of the original coefficients $b$ that correspond to particular combinations of conditions that you want to compare. Look at the net difference between the conditions you want to compare, and only then exponentiate.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you for the good help. So for summer in bays e^(b) is e^(-0.99)+(-0.76)+(1.11) = 0.64. I appreciate it. $\endgroup$
    – Steve
    Commented Jan 4, 2022 at 0:07
  • $\begingroup$ @Steve you missed some parentheses but you have the general idea; it's e^(-0.64) = 0.52 That's the ratio with respect to the reference condition of Channel/Spring. If you want actual estimated counts for a condition you need to include the intercept. It's best not to focus on the individual coefficients but rather examine differences between particular conditions of interest, and let the software perform the calculations along with corresponding confidence intervals. $\endgroup$
    – EdM
    Commented Jan 4, 2022 at 15:43
  • $\begingroup$ Thanks for the clarification $\endgroup$
    – Steve
    Commented Jan 6, 2022 at 18:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.