Understanding Output of ur.df Test: What Do the z.diff.lag# Indicate?

Question

When reading the output of summary(ur.df(ts)) in R, what do the z.diff.lag# coefficients indicate?

Are they error terms for “at” in the Dickey-Fuller regression expression? For example:

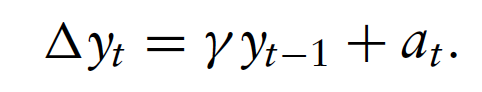
To help with instruction, I'll generate some stationary data and run the ur.df function with summary wrapped around it.

# generate white noise
n <- 200
x <- rnorm(n, 
           mean = 0, 
           sd = 1)
# make into time series object
y <- ts(x)

Now, for example, I run an ADF test to check if my time series is not a random walk masquerading as stationary data.

For the ADF test, because the data is white noise (with no drift or trend), I leave the ur.df test type="none".

To generate lots of z.diff.lag# coefficients, I will run the ADF test at 5 lags:

summary(ur.df(y,
              type = c("none"),
              lags = 5))

And here is the output:

## 
## ############################################### 
## # Augmented Dickey-Fuller Test Unit Root Test # 
## ############################################### 
## 
## Test regression none 
## 
## 
## Call:
## lm(formula = z.diff ~ z.lag.1 - 1 + z.diff.lag)
## 
## Residuals:
##      Min       1Q   Median       3Q      Max 
## -1.82322 -0.81705 -0.08905  0.62597  2.30963 
## 
## Coefficients:
##             Estimate Std. Error t value Pr(>|t|)    
## z.lag.1     -1.04664    0.17779  -5.887 1.78e-08 ***
## z.diff.lag1  0.02807    0.15987   0.176    0.861    
## z.diff.lag2  0.11422    0.14319   0.798    0.426    
## z.diff.lag3  0.06688    0.12474   0.536    0.593    
## z.diff.lag4  0.07115    0.10538   0.675    0.500    
## z.diff.lag5 -0.01979    0.07381  -0.268    0.789    
## ---
## Signif. codes:  0 '***' 0.001 '**' 0.01 '*' 0.05 '.' 0.1 ' ' 1
## 
## Residual standard error: 0.9941 on 188 degrees of freedom
## Multiple R-squared:  0.5251, Adjusted R-squared:   0.51 
## F-statistic: 34.65 on 6 and 188 DF,  p-value: < 2.2e-16
## 
## 
## Value of test-statistic is: -5.8871 
## 
## Critical values for test statistics: 
##       1pct  5pct 10pct
## tau1 -2.58 -1.95 -1.62

Lo-and-behold, the data is stationary. No surprises.

In the summary(ur.df(ts)) results, I understand the t value for z.lag.1 is the test-statistic value at lag 5. I also understand the p-value for this coefficient and what it means for the Critical values.

But what I do not understand is the series of five z.diff.lag# coefficients. My only thought is these are error terms (the “at” values) for the Dickey-Fuller algebraic equation. But again, I do not understand what the p-value means for these five z.diff.lag# indicate.

Any insight is much appreciated.

Answer 1

In an augmented Dickey-Fuller test, the model equation (for type=trend specification) is $$ \Delta y_t = \alpha + \beta t + \gamma y_{t-1} + \delta_1\Delta y_{t-1} + \dots + \delta_{p-1}\Delta y_{t-p+1} + \varepsilon_t $$ (I used the notation from Wikipedia) where you may set $\beta=0$ (type=drift) and also $\alpha=0$ (type=0). The lagged first differences of $y_t$ are there to account for any autocorrelation in $\Delta y_t$, as $\Delta y_t$ could be e.g. an ARMA process. ur.df uses z instead of $y$, so z.diff.lagj corresponds to $\Delta y_{t-j}$. The corresponding coefficients, $p$-values and such characterize these lagged first difference terms in the model.