If the p-value suggests significance, can the test statistic give any more information? I.e., can you use the test statistic to tell if some of the significance is more significant than other parts?
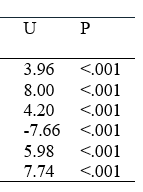
I ran Mann-Whitney U tests and these U's are, overall, far higher than any other U's in my analysis, even for other tests that resulted in p<.001. Is it valid to say that the test which resulted in the 8.00 or -7.66, for instance, were more significant than others, given that no other U statistic of the 48 tests I ran even exceeded 5.00?
Edit: In response to Glen_b's comment, I relooked at my output and sure enough Mann-Whitney U is a separate value from the (standardized) test statistic.
I suppose, then, my question hasn't changed, because I'm still asking about the test statistic, just properly labelled now. What am I supposed to call those values in my write-up?