1
$\begingroup$

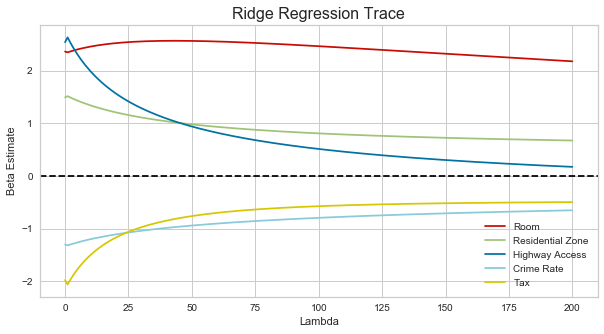
From my understanding, Ridge regression tends to shrink coefficients towards 0 as lambda increases. However, it seems this is not always the case - for features which are more statistically significant, Ridge Regression can increase their coefficient as lambda increases. Why is this? And how does the Ridge penalty cause this?

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ A related question is stats.stackexchange.com/questions/414088 would my answer there answer your question here? "The ridge path is not a straight line ... Shrinking the parameters in a straight line from the OLS solution to 0, means that the sum of squared error becomes high. Taking a detour allows to shrink the parameters with less reduction of the sum of least squared error." $\endgroup$
    – Sextus Empiricus
    Commented Nov 17, 2022 at 7:36

0

Reset to default