Causal modeling in the presence of a latent variable

Question

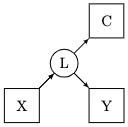
Suppose that four variables of $X$, $Y$, $L$, and $C$ have the following relationships in the form of directed acyclic graph.

$X$, $Y$, and $C$ are observable variables while $L$ is a latent (unobservable) variable. If one is interested in modeling the relationship between $X$ and $Y$ through, for example, regression, is it reasonable to include $C$ as a covariate and condition the $X$-$Y$ association on $C$? Alternatively, how about when one is interested in the association between $C$ and $Y$?

Answer 1

In the first scenario, C is a descendant of a mediator, so contrary to previous answers, yes it would be harmful to include if your interest is in estimating the total effect of X on Y. See DAG 12 here:

http://causality.cs.ucla.edu/blog/index.php/2019/08/14/a-crash-course-in-good-and-bad-control/

Agree with earlier answers that there's no available strategy to identify the effect of C on Y given this DAG is correct.

Answer 2

In your first scenario, you certainly can include $C$ on the RHS of your linear regression model, but you shouldn't need to if your DAG is correct. If your DAG is correct, then $C$ does not cause $Y$ in any way, and is not a confounder (does not set up a back door path). All of the causal effect of $X$ on $Y$ is mediated through $L:$ that's fine. There's nothing special you need to do, just regress $Y$ on $X.$

In your second scenario - finding the association between $C$ and $Y$ - you have a problem: $L$ is a confounding variable. Moreover, if you have no other variables present anywhere, then I don't see a way to estimate the causal effect of $C$ on $Y.$ $X$ is not an instrument, because if it were, it would have be pointing into $C$ and nothing else. You're not even really sure that $C$ has a causal effect on $Y.$ Technically, of course, your DAG says it doesn't have a causal effect on $Y.$ I'd say you're stuck, here.