In the Definition 3.4.1 of Pearl's causal inference book (Primer), the second rule for the front door criterion is "There is no backdoor path from $X$ to $Z$". But from my understanding, there EXISTS one backdoor path from $X$ to $Z$: $X \leftarrow U \rightarrow Y \leftarrow Z$. And this backdoor path is blocked by the collider $Y$.
Can anyone help me understand this rule? Thank you.
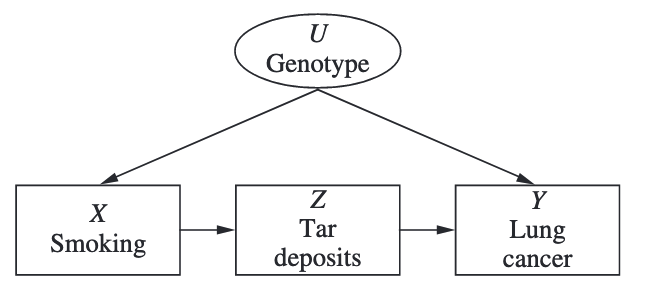
I can understand your confusion. In the Front-Door Criteria of Definition 3.4.1, the original text for #2 read, "There is no unblocked path from $X$ to $Z$." The book's errata changed that to "There is no backdoor path from $X$ to $Z$." I admit it's a bit confusing either way: Pearl was a little more careful in his 2009 book Causality: Models, Reasoning, and Inference, 2nd Ed. On page 82 of that book, Definition 3.3.3 (Front-Door), the second item on the list reads: "there is no unblocked back-door path from $X$ to $Z;...$"
It certainly is the case that $Y$ satisfies the Backdoor Criterion (Def. 3.3.1 on page 61) relative to $(X,Z).$ This is what enables Pearl to say, in the derivation of the Front-Door criterion, that
$$P(z|\operatorname{do}(x))=P(z|x).$$
That plus Def. 3.4.1, #1 and #3 are sufficient to enable the Front-Door procedure to work.
