6
$\begingroup$

I'm reading Causal Inference in Statistics: A Primer by Pearl, et. al. and I'm a little confused by the definition of front door. The definition in the book (Definition 3.4.1) is:

A set of variables, Z is said to satisfy the front-door criterion relative to an ordered pair of variables (X, Y) if:

  1. Z intercepts all directed paths from X to Y
  2. There is no unblocked path from X to Z
  3. All backdoor paths from Z to Y are blocked by X.

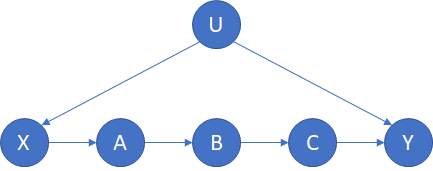
I understand the first and third requirements. But, the second criteria, I'm a little confused. Here is the situation which confused me. Let's say my causal graph looks like this:

front door criteria question

So, let's say I set Z = {B}. This satisfies criteria 1 (since the only path from X to Y goes through B. This also satisfies criteria 3 (since the only back-door path from B to Y is B <-- A <-- X <-- U --> Y and this is blocked by X.

But does it satisfy criteria 2? I think the answer is no because there is an unblocked path from X to Z namely X --> A --> B. As such, my understanding is that I need to make Z = {A, B} to satisfy all three criteria. Or am I missing something and do I need to make Z = {A, B, C}?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
6
$\begingroup$

Criterion 2 is written wrong. It should be:

  1. there is no unblocked back-door path from X to Z

In your diagram, there is an unblocked path from X to Z, but it is not a back-door path, which is a path between X and Z that contains an arrow into X.

Improve this answer
$\endgroup$
7
  • $\begingroup$ It wouldn't have to backdoor; if X and Z both pointed to a conditioned-upon collider, then there would be an unblocked, non-backdoor path that would indeed violate the frontdoor criterion. $\endgroup$
    – Noah
    Commented Apr 9, 2019 at 22:34
  • $\begingroup$ @Noah Are you sure about that? The criterion I gave is a direct quote from Pearl's 2nd edition textbook (Definition 3.3.3 on p. 82) $\endgroup$
    – Jake Westfall
    Commented Apr 10, 2019 at 2:19
  • $\begingroup$ Not sure, but the situation I described would yield the same pattern of association as an unblocked backdoor path, so that's why I thought to add it. $\endgroup$
    – Noah
    Commented Apr 10, 2019 at 3:36
  • $\begingroup$ @Jake Westfall thanks. So, in the example I gave, I could set Z to any non-empty subset of {A, B, C} right? $\endgroup$
    – roundsquare
    Commented Apr 10, 2019 at 12:06
  • 2
    $\begingroup$ Yes, although the estimation formula would further simplify with two or more elements from {A, B, C}, because of d-separation. @JakeWestfall 's answer is correct, see for example Pearl's "Causality" book, 2nd edition, Definition 3.3.3. $\endgroup$
    – Julian Schuessler
    Commented Apr 10, 2019 at 12:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.