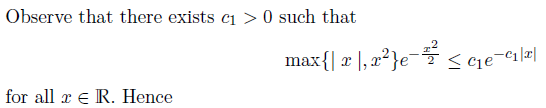I am reading a book in which the proof of Expectation of normal is 0 is proved. I couldn't figure out how the right hand integral of equation 6.1.2 is less than infinity and how c1 is exactly chosen? Any help is appreciated
4
-
1$\begingroup$ Where is this from ? It is in fact not true that $$\int_{-\infty}^{\infty}e^{-c_1 x} <\infty$$ You'd need to replace $x$ by $|x|$ for it to be true. $\endgroup$– Stratos supports the strikeCommented Oct 2, 2023 at 11:43
-
$\begingroup$ Can you explain your statement $\endgroup$– JayCommented Oct 2, 2023 at 11:51
-
1$\begingroup$ If $c_1>0$, then $e^{-c_1 x}\to \infty$ as $x\to-\infty$, so the integral $\int_{-\infty}^0 e^{-c_1 x} dx$ is trivially divergent. $\endgroup$– Stratos supports the strikeCommented Oct 2, 2023 at 11:54
-
1$\begingroup$ I found a pdf version of this textbook in which (6.1.2) is correctly rendered: in particular, it shows that "$e^{-c_1 x}$" is intended to be "$e^{-c_1|x|}$" (a function of the absolute value of $x$). $\endgroup$– whuber ♦Commented Nov 25, 2023 at 15:57
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
In one (pdf) copy of this passage the assertion instead is
There exists $c_1\gt 0$ such that $f(x) = \max\{|x|,x^2\}e^{-x^2/2}\le c_1 e^{-c_1 |x|}$ for all $x\in \mathbb R.$
Here is a screen shot:
In this version, "$-c_1 x$" is "$-c_1|x|.$" Nevertheless, the assertion is false.
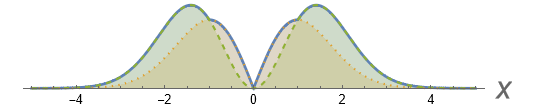
A good way to study arguments like this is to plot the functions involved. Here are the graphs of $|x|e^{-x^2/2}$ (dotted orange), $x^2 e^{-x^2/2}$ (dashed green), and their maximum (solid blue).
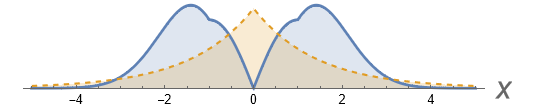
What the text intends to do is bound the first two curves above by a curve whose area is easy to find and is finite. That cannot be done using curves of the form $c \exp(-c |x|).$ The best any curve of this form can do at the points where $f$ is maximized is shown in orange here:
It's not even close to covering the graph of $f.$
The problem is easily fixed by considering an even simpler comparison curve, such as $c \exp(-|x|)$ for some value of $c$ to be found. A little Calculus will show that $c=4$ (barely) works and any larger value of $c$ therefore works with room to spare:
The area under the dashed orange (bi-exponential) curve is $8.$ Consequently, the areas under the graphs of $|x| \exp(-x^2/2)$ and $x^2\exp(-x^2/2)$ must each be less than $8.$ Because $8$ is finite, the point is made.
