Mixed signs of coefficient in mediation - what does that mean in fact?

Question

I have an interpretation problem that I have not been able to solve using any of the books I have found on mediation. I understand many aspects of it, but the model in which the signs are mixed up gives me a problem with interpretation - that is, with correctly understanding the true essence of this mediation.

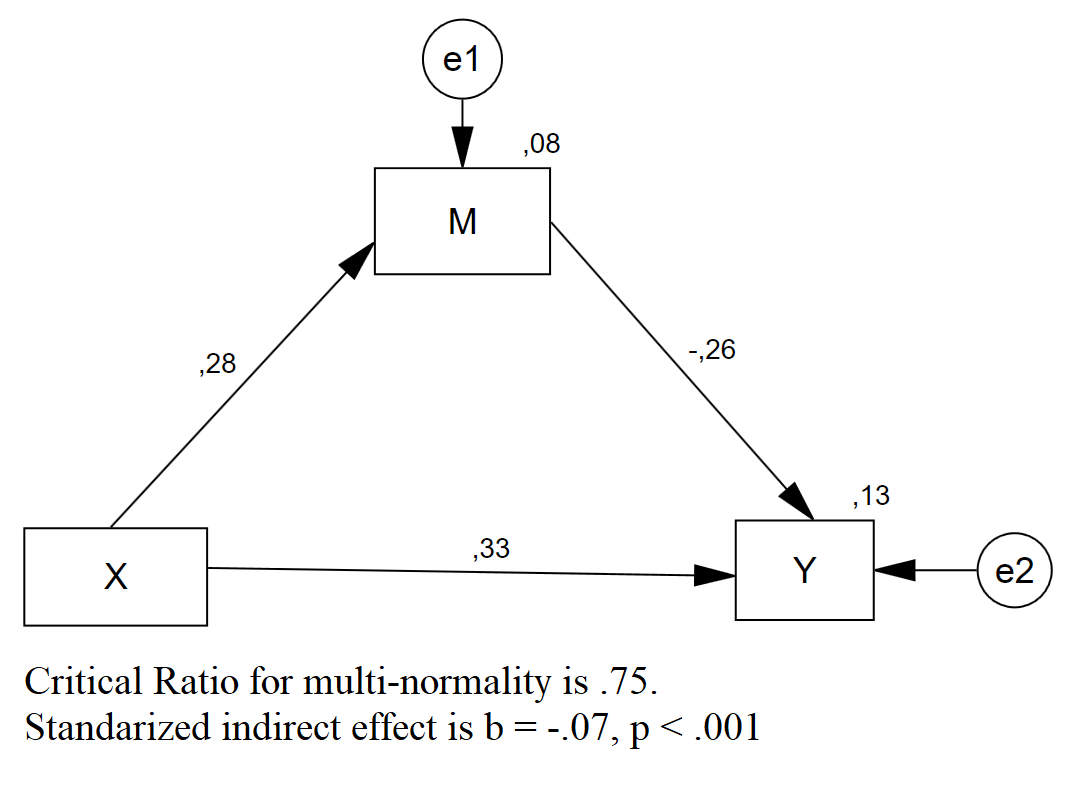
Consider the following model. I'm attaching the data here. We have three quantitative variables: the independent variable X, the dependent variable Y and the mediator M.

Let's start with conducting mediation using the classic Baron/Cohen/Kenny method. We have partial mediation, the Sobel Z test is significant. We can talk about mediation. Here's the result:

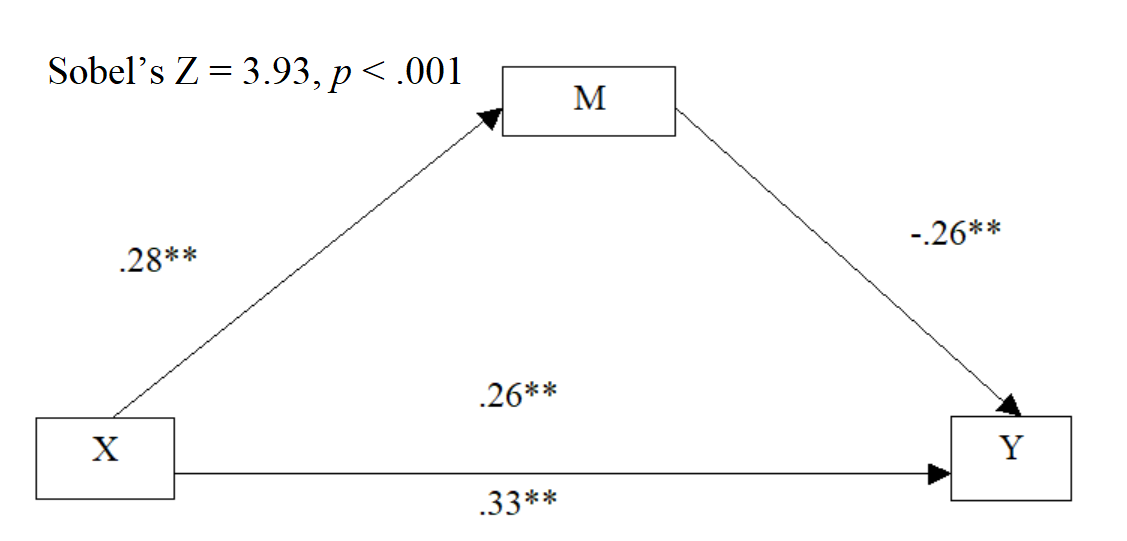
We can obtain the same result using SEM modeling in AMOS/R, where we obtain the same standarized path coefficients. The bootstrap method can be used to obtain the value of the path coefficient of the indirect influence path, as well as its statistical significance. Again, we can speak of mediation.

It's weak, I know it can be considered a negligible artifact in some cases, but I also know that if I dug into the data I could easily increase the strength of this effect, so that's not the point.

I am not satisfied with the general answers I have found so far which simply interpret the signs without understanding what is happening inside the mediation and how to properly understand the effects. Here's what's incomprehensible to me:

1. When all signs are the same, we can roughly understand what happens under the influence of the mediator. But in this case, a strange contradiction appears. The independent variable X increases the level of Y directly, but (taking into account the sign of the indirect effect) simultaneously decreases its level through the mediator M. Do I understand this correctly?
2. If this is the case, then what is actually happening with the Y variable? We can probably read from the difference in the standardized coefficients what is a stronger predictor of the Y variable (and therefore whether it decreases or increases more), but if the b coefficients were more similar, do we have any idea what is happening with the Y variable in such a conglomeration of variables? In addition, taking into account the result of the classical model, the mediator seems to increase the level of influence of X on Y, but the sign of the mediator is negative!
3. How do we know about mediation or suppression at all (in this case)? In the case of the same signs, we know about mediation because the predictor changes the "strength" of the standardized coefficient in the regression equation, but here we have two contradictory influences at the same time. How on earth does mediation "know" that it is mediation (in this case)?
4. Is there a standard interpretation of such effects? Maybe one should simply move on to other analyses and such a result is just a hint for a more non-standard approach? I see a slight curvilinear relationship in the three-dimensional projections, but the effects of interactions in subgroups do not come out statistically significant. Is there any other way/thing that should be taken/tested now?
5. Does the mediator increase/decrease the level of the dependent variable, or do its mathematical properties only lower our observation of the effect of X on Y? That is - in fact, the level of influence of X on Y does not change, the strength of the influence of the independent variable remains unchanged, the only thing that actually happens is an artificial lowering of the standardized coefficient, thanks to which we observe mediation.

Thank you in advance for all your suggestions and joint attempts to work on this problem. Of course, I will be happy to read the literature and articles You'll recommended.

Answer 1

1. Yes.

2. Not sure how to answer.

3. Yes, this looks like suppression. That's what suppression is. The model don't "know" that it is mediation. The model is trying to make sense of the data that you have given it, the model is not "true" in any sense - if this is the correct data generating model, then these are the parameters.

4. This is a suppressor. Suppressors are interesting if you can interpret them, and annoying if you can't.

Think about what this would mean if you weren't doing mediation, but a regular regression. You first regress Y on X. The effect is low. You then regress Y on X and M, the X effect is increased, the M effect is negative.

In Horst's original example, if we think about it in mediation terms (which he, as far as I know, didn't) pilot ability (X) was uncorrelated with verbal ability (Y), but when you add in technical ability you find a negative effect of verbal ability and a positive mediation effect.

Because you need verbal ability to pass the technical ability test, verbal ability gives you an unfair boost. If you are technically good, and verbally poor, your technical score is biased, and your technical ability must be very good. If you are technically and verbally good you have almost cheated the technical test by being verbally good. In choosing between two pilots with an equally high technical score, you should choose the one with the lower verbal score.

5. It's hard to make these statements about whether a variable increases the value of Y, because it's not clear if you are controlling for X or not. I'm not sure I would call the change in the coefficient "artificial" (but these are the kinds of problems where you need precise language - and it's hard to map the language of everyday life onto this sort of problem.)