I have a data set with 561 observations. I want to compare two groups on an outcome measure with 3 categories. I have a couple of covariates.
I used the following syntax (Stata SE 12.1):
mlogit outcome_measure covariate_1 covariate_2 i.covariate_3 i.covariate_4 group
To check for Independence from Irrelevant Attributes (IIA) I used
mlogtest , haus
This gave me the following output:
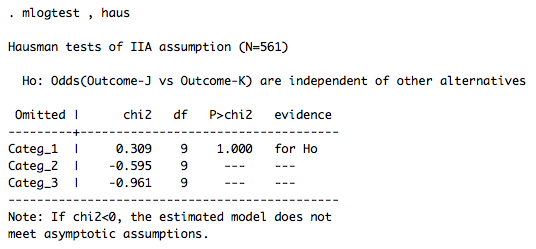
I understand that this means that the data do not meet the IIA assumption.
Stata then suggests to use the suest (Seemingly Unrelated Estimation) command. I could try this, but I am not sure if this is a valid alternative. What do you guys think?
EDIT (2013-09-18):
@GUNG: I asked one of my statistics professors. He said that the additional tests won't be performed if the assumption is not violated for one of the outcomes (in the case of three outcome categories). He therefore thinks that I can proceed with the mlogit model.
@Dimitriy V Masterov
I ran the Small-Hsiao IIA test. These are the outcomes:
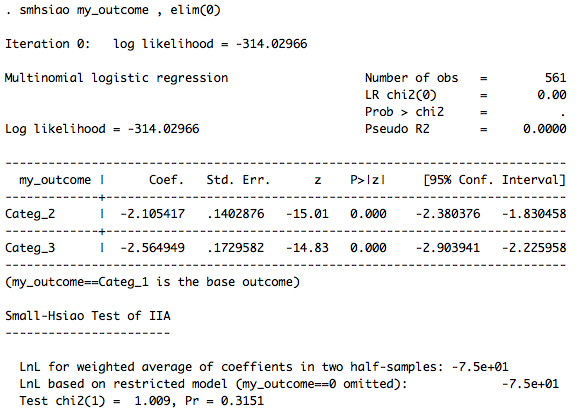
I guess this means IIA is not violated. Do you agree?
EDIT (2013-09-22):
As Dimitriy V. Masterov suggested, I ran the new syntax for Hausman. Because I have 3 categories in my outcome variable, I compared the Full model to all models with one category of my outcome variable omitted (Full vs. 1+2, Full vs. 1+3, Full vs. 2+3). These are the outcomes:
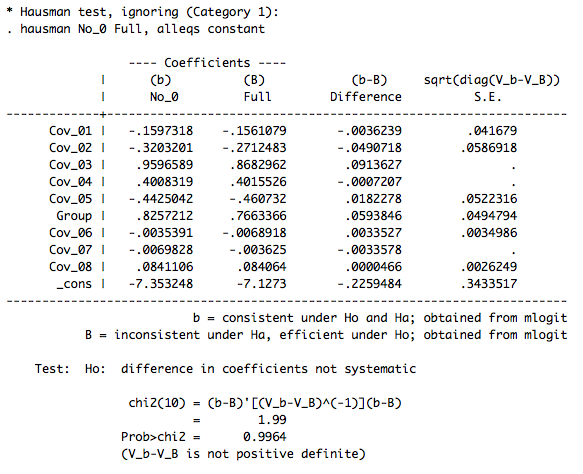

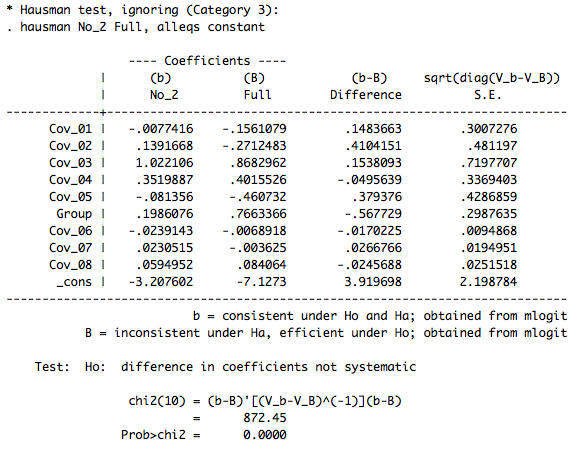
It looks like leaving out the third category changes the model significantly. Does this mean that I cannot use Multinomial Logistic Regression and that I should move to (the suggested) suest (Seemingly Unrelated Estimation)?
suest is giving the following outputs:
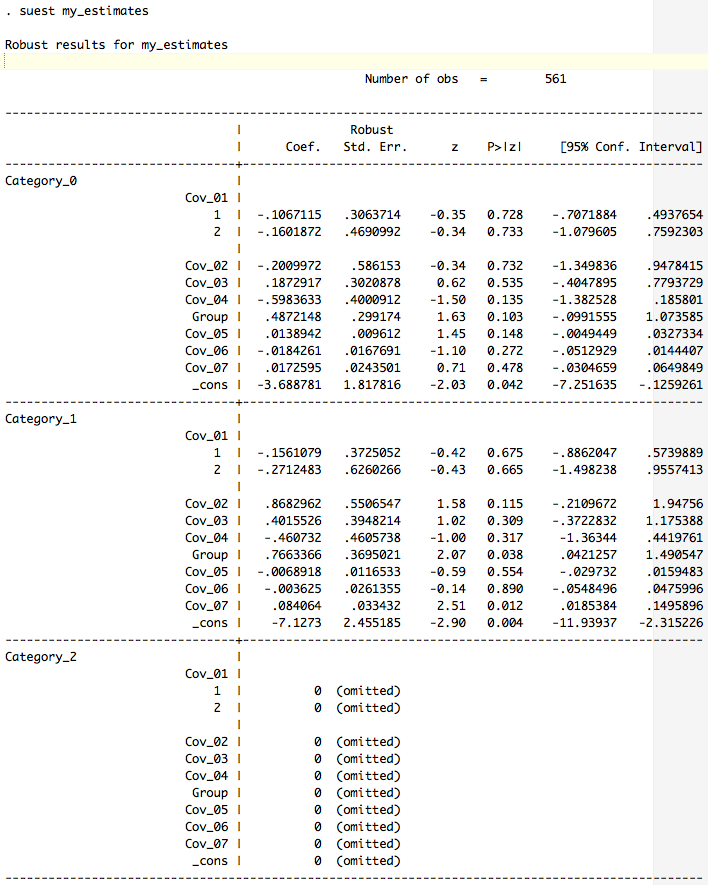
The output looks fine to me and it supports my hypothesis, but I am not sure if suest is valid, what it assumptions are and how I can test these.
Any comments are welcome!

Categ_1is non-significant, and the other two, having negative values for thechi2, must not "meet asymptotic assumptions". Thus, I'm not sure that you can validly conclude that "the data do not meet the IIA assumption". Possibly nothing can be concluded relative to the IIA assumption. Instead, I think you want to find out what the asymptotic assumptions of the Hausman test are, and what it is about your data that violate them. $\endgroup$