Reference with distributions with various properties

Question

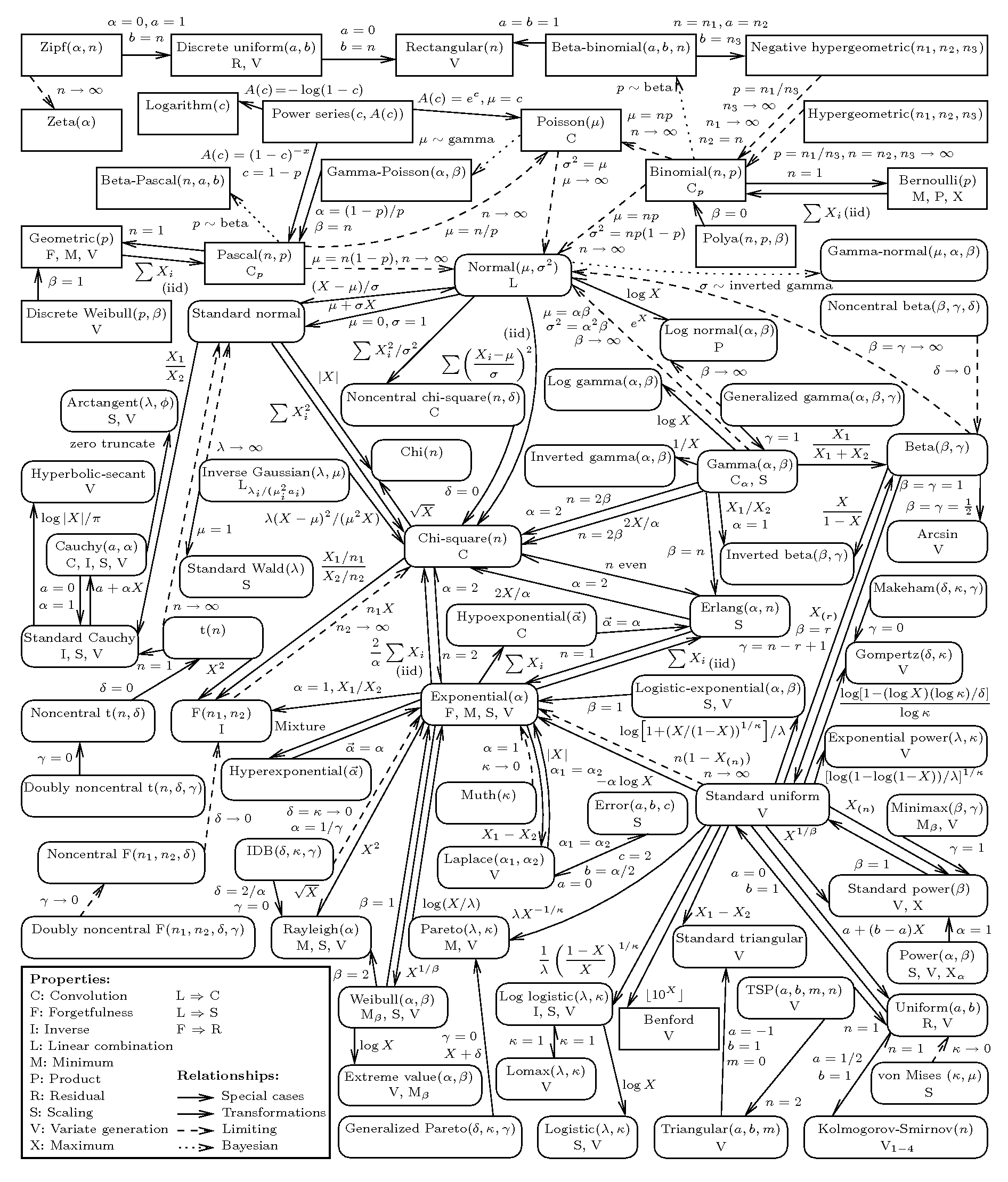
I often find myself asking questions like, "I know this variable $x$ lies in $(0,1)$ and most of the mass lies in $(0,.20)$ and then declines continuously towards 1. What distribution can I use to model it?"

In practice, I wind up using the same few distributions over and over again simply because I know them. Instead, I'd like to look them up in a more systematic way. How do I go about accessing the wealth of work that probabilitists have done developing all of these distributions?

Ideally I'd like a reference organized by properties (region of support, etc.), so I can find distributions by their characteristics and then learn more about each distribution based on the tractability of the pdf/cdf and how closely the theoretical derivation fits the problem I'm working on.

Does such a reference exist, and if not, how do you go about choosing distributions?

Answer 1

The most comprehensive collection of distributions and their properties that I know of are

Johnson, Kotz, Balakrishnan: Continuous Univariate Distributions Volume 1 and 2;

Kotz, Johnson, Balakrishnan: Continuous Multivariate Distributions;

Johnson, Kemp, Kotz: Univariate Discrete Distributions;

Johnson, Kotz, Balakrishnan: Multivariate Discrete Distributions;

The books have a broad subject index. All books are from Wiley.

Edit: Oh yes and then there also is the nice poster displaying properties and relationships between univariate distributions. http://www.math.wm.edu/~leemis/2008amstat.pdf This might be of further interest.

Answer 2

honestly, there are way too many distributions that I have no idea about. I do believe however that knowing them is not an asset, one must know how to use them. Anyway, back to your question, I always find this diagram quite informative and useful, it's like probability distributions cheatsheet.

http://jonfwilkins.com/wp-content/uploads/2013/06/BaseImage.png

Answer 3

No book could cover all distributions, as it is always possible to invent new ones. But

Statistical distributions by Catherine Forbes et al. is a concise book covering many of the more commonly used distributions

while

A primer on statistical distributions by N. Balakrishnan and V.B. Nezvorov

is also fairly concise, but rather more mathematically oriented.

The nearest approach to a treatise is the series started by N.L. Johnson and S. Kotz, being continued by A.W. Kemp and N. Balakrishnan, and currently published by John Wiley.

This isn't a complete list even of surveys of distributions, but Googling your local Amazon site easily gets you other ideas.

Answer 4

Merran Evans, Nicholas Hastings, Brian Peacock - Statistical distributions - John Wiley and Sons

I have the second edition and the distributions are in simple alphabetical order (from Bernoulli to Wishart central distribution).

Answer 5

The Hand-book on Statistical Distributions for Experimentalists by Christian Walck at the University of Stockholm is pretty decent....and FREE!! It covers over 40 distributions from A to Z, with each distribution described with its formulas, moments, moment generating function, characteristic function, how to generate a random variate from this distribution, and much more. Very nice for a free pdf.

Answer 6

Ben Bolker's "Ecological Models and Data in R" has a section "bestiary of distributions" (pp 160-181) with descriptions of the properties and applications of many common and useful distributions.

It is written at the level of a grad level course in ecology, so it is accessible to non-statisticians. Less dense than the Johnson, Kotz et al references in the answer by @Momo, but gives more practical details than a list or appendix might.

Answer 7

The Loss Models by Panjer, Wilmot and Klugman contains a good appendix regarding distribution pdf, their support and parameter estimation.

Answer 8

A study of bivariate distributions cannot be complete without a sound background knowledge of the univariate distributions, which would naturally form the marginal or conditional distributions. The two encyclopedic volumes by Johnson et al. (1994, 1995) are the most comprehensive texts to date on continuous univariate distributions. Monographs by Ord (1972) and Hastings and Peacock (1975) are worth mentioning, with the latter being a convenient handbook presenting graphs of densities and various relationships between distributions. Another useful compendium is by Patel et al. (1976); Chapters 3 and 4 of Manoukian (1986) present many distributions and relations between them. Extensive collections of illustrations of probability density functions (denoted by p.d.f. hereafter) may be found in Hirano et al. (1983) (105 graphs, each with typically about five curves shown, grouped in 25 families of distributions) and in Patil et al. (1984).

This is from Chapter 0 of a book on continuous bivariate distributions, which provides an elementary introduction and basic details on properties of various univariate distributions. I remember I enjoyed reading Ord (1972) very much, but I can't now remember why.

Answer 9

The series of books by Johnson, Kotz & Balakrishnan (edit: which Nick has also mentioned; the original books were by the first two authors) are probably the most comprehensive. You probably want to start with Continuous Univariate Distributions, Vols I and II.

A couple more:

Evans, Hastings & Peacock, Statistical Distributions

Wimmer & Altmann, Thesaurus of univariate discrete probability distributions

There's also many other books, sometimes for more specialized applications.