How can you derive the sample size to obtain a particular type II error rate?

Question

I often see that the sample size for a $z$-test to achieve a particular type II error rate $\beta$ (at a given significance level, $\alpha$) is:

$$n = \frac{(Z_{1-\alpha/2}+Z_{1-\beta})^2\sigma^2}{\delta^2}$$

How do they reach that conclusion/determine that?

Answer 1

Here's an outline of the basic ideas:

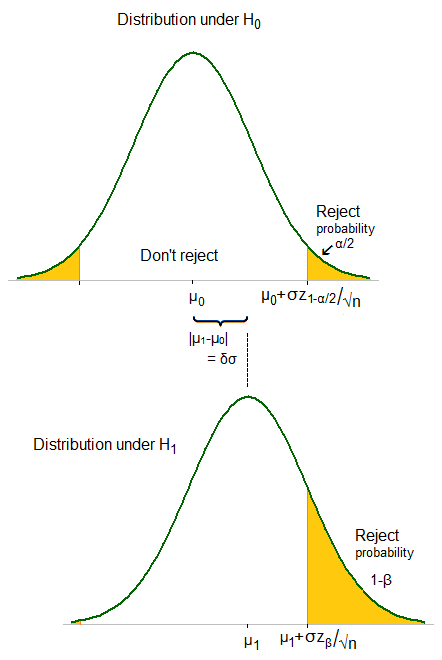
The same critical value, $C$, applies whether $H_0$ is true or $H_1$ is true.

$C=\mu_0+\sigma Z_{1-\alpha/2}/\sqrt{n}$

$C=\mu_1+\sigma Z_\beta/\sqrt{n}$

$\mu_0+\sigma Z_{1-\alpha/2}/\sqrt{n}=\mu_1+\sigma Z_\beta/\sqrt{n}$

$(\mu_1-\mu_0)/(\sigma/\sqrt{n}) = (Z_{1-\alpha/2}- Z_\beta)$

$[(\mu_1-\mu_0)/\sigma]\sqrt{n} = (Z_{1-\alpha/2}+ Z_{1-\beta})$

$\sqrt{n} = (Z_{1-\alpha/2}+ Z_{1-\beta})/[(\mu_1-\mu_0)/\sigma]$

and then you just square both sides

$n = (Z_{1-\alpha/2}+ Z_{1-\beta})^2/[(\mu_1-\mu_0)^2/\sigma^2]$

$\quad = (Z_{1-\alpha/2}+ Z_{1-\beta})/\delta^2$

Note: (1) Here I'm doing just the case $\mu_1>\mu_0$. To cover both possibilities, we really need $|\mu_1-\mu_0|$ where I have $(\mu_1-\mu_0)$; the outcome is the same.

$\quad\quad$(2) This ignores a small piece of area to the far left of the second diagram.

$\quad\quad\quad\,\,\,$When $\delta$ is large, this is negligible.