I am studying the definition of leverage, and I understand it in terms of formulas. However, if I would have a plot like this for instance, how could I see which of these points has the highest leverage? Which one would it be in this plot for instance?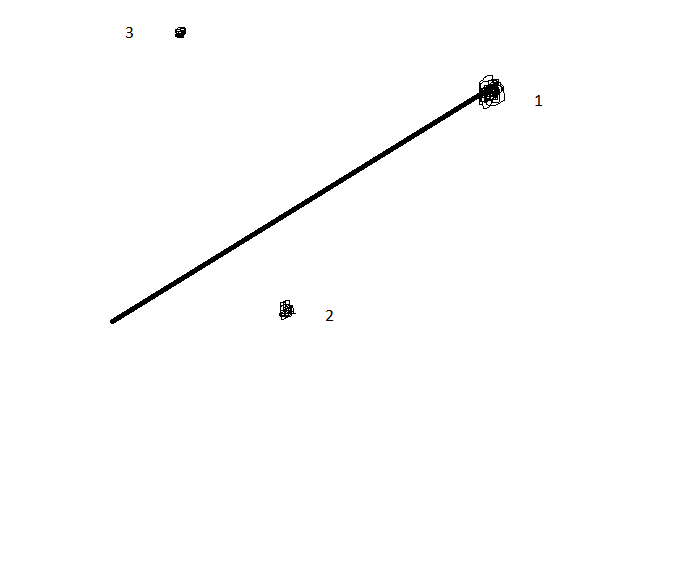
$\begingroup$
$\endgroup$
19
-
1$\begingroup$ @john It is a plot that I drew myself (as you can see). There is not really any work that I can do. I read something about leverage and thought of this question. $\endgroup$– student330247324Commented Jul 8, 2014 at 13:52
-
4$\begingroup$ You seem to miss the point. There must be more x-values than the three you drew (the line you drew is not remotely near the least squares line for those three points), and so we need to know where the mean of all the x-values is, as whuber already said. Without that information, there's no way to say which has the higher leverage. $\endgroup$– Glen_bCommented Jul 8, 2014 at 15:34
-
1$\begingroup$ @Glen_b Yes I do. The leverage is defined as $h_{ij}=\frac{1}{n}+\frac{(x_j-\bar{x})(x_i-\bar{x})}{\sum (x_i-\bar{x})^2}$ (can be derived from the hat matrix). $\endgroup$– student330247324Commented Jul 9, 2014 at 9:32
-
1$\begingroup$ The relevant definition in this problem is $h_{ii}$: en.wikipedia.org/wiki/Leverage_%28statistics%29#Definition . Note that all points will have the same denominator. Which point will have the largest numerator? $\endgroup$– Glen_bCommented Jul 9, 2014 at 9:37
-
1$\begingroup$ Yes, distance from the mean is the critical issue (for univariate $x$ at least). You may like to review whuber's initial comment, which was attempting to point you there without flat out stating it. I can't say for certain what the ordering is because your diagram still isn't sufficiently clear about the precise location of $\bar{x}$. $\endgroup$– Glen_bCommented Jul 9, 2014 at 9:49
|
Show 14 more comments
1 Answer
$\begingroup$
$\endgroup$
4
The leverage is $h_{ii}=\frac{1}{n}+\frac{(x_i-\bar{x})^2}{\sum (x_i-\bar{x})^2}\,$.
The term $\frac{1}{n}$ and the denominator of the second term $\sum (x_i-\bar{x})^2$ are the same for every $i$, so the point with the largest $(x_i-\bar{x})^2$ has the highest leverage.
This means that the point furthest from the mean has the highest leverage.
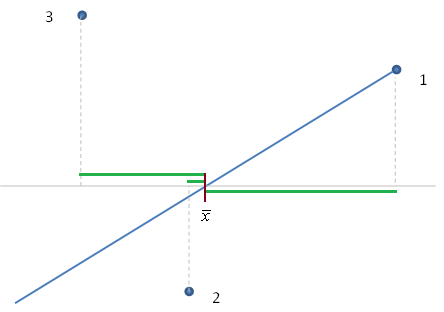
In the diagram, point 1 is the furthest from $\bar x$ in the x-direction, so it will have the largest leverage of the three points.
-
$\begingroup$ Thanks again. But isn't point 1 the farthest away from $\bar{x}$? $\endgroup$ Commented Jul 9, 2014 at 11:42
-
$\begingroup$ Correct. I was writing without being able to see the plot and misremembered which point carried which label. Fixed now, thanks. $\endgroup$– Glen_bCommented Jul 9, 2014 at 12:09
-
1$\begingroup$ +1 I love illustrations, especially those that could stand on their own as solutions (even absent the words). But in this case wouldn't that suggest drawing horizontal dashed lines between the indicated points and the vertical line through $\bar{x}$? The vertical dashed lines appear to be relevant only by virtue of being directly proportional to those horizontal distances. $\endgroup$– whuber ♦Commented Jul 9, 2014 at 15:48
-
1$\begingroup$ @whuber The vertical dashed lines weren't themselves to indicate distance from the mean, but were intended to make the the abscissa for each point explicit, at which point the horizontal distance from $\bar x$ is easy to judge. But you're right that those distances should be explicitly marked. I'll do that now $\endgroup$– Glen_bCommented Jul 9, 2014 at 22:34