2
$\begingroup$

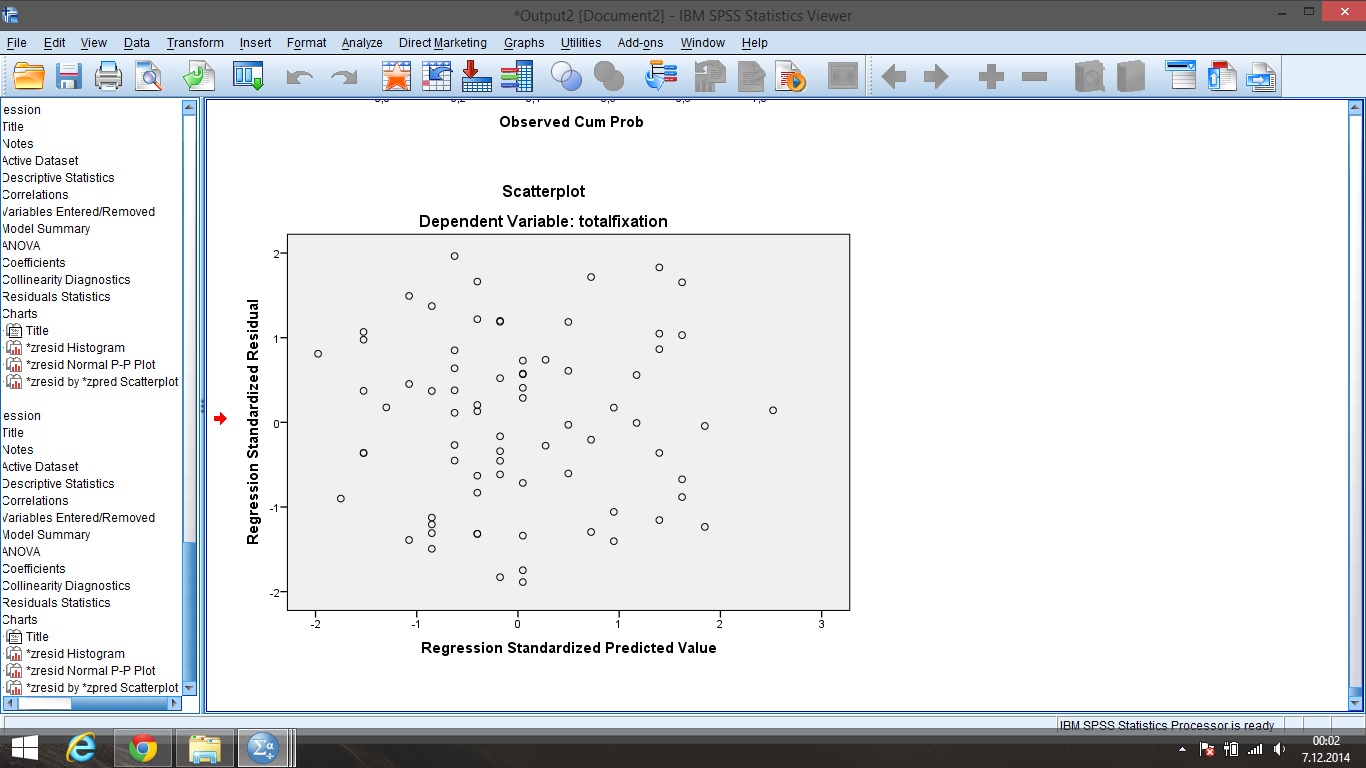
I have 2 continuous variables and I want to conduct a simple linear regression, 1 DV and 1 IV. There is a moderate correlation between them. However, I suspect there may be some heteroscedasticity and I cannot run a regression in SPSS. Also I am not sure if my residual scatter plot really shows a triangle / heteroscedasticity. I have 75 participants. Here is my scatter plot. Any suggestions?

enter image description here

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ I don't really see a triangle here. What makes you think you have heteroscedasticity? The predicted values seem to come in regular intervals / at discrete locations, do you know why that is? Were the original data grouped at fixed intervals on X? $\endgroup$
    – gung - Reinstate Monica
    Commented Dec 6, 2014 at 22:09
  • $\begingroup$ So as i have no heteroscasticity, cant i make linear regression? $\endgroup$
    – Emrah Dolgunsöz
    Commented Dec 6, 2014 at 22:26
  • 1
    $\begingroup$ Note that the existence of Heteroscedacity will not effect the coefficient estimates in the regression but rather only the standard errors of the coefficients. So your estimates from a linear regression will be the same regardless of adjustment. I suppose I can see a "football" like shape to the residual plot which can be a mark of heteroscedacity in certain data. I am not familiar with SPSS but most statistical packages offer a "robust" option in regression which adjusts for heteroscedacity in standard error. $\endgroup$
    – Zachary Blumenfeld
    Commented Dec 6, 2014 at 23:58
  • 1
    $\begingroup$ @gung has it right: heteroscedasticity. Show us the scatter plot too, but there is little to worry about in that plot in my view. $\endgroup$
    – Nick Cox
    Commented Dec 7, 2014 at 1:58
  • $\begingroup$ Thanks a lot, so it seems i accomplished all assumptions for linear regression. This site is awesome! $\endgroup$
    – Emrah Dolgunsöz
    Commented Dec 7, 2014 at 10:53

1 Answer 1

Reset to default
1
$\begingroup$

From your plot, I don't think there is problematic heteroscedasticity. I think you are OK to run a simple linear regression here.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.