Be $X\sim N(\mu,1)$ and $Y\sim Inverse-Gamma(\alpha,\beta)$.
For the Inverse-Gamma, I usually use the parameterization which leads to the following probability distribution function for Y:
$f(y;\alpha,\beta)=\frac{\beta^{\alpha}}{\Gamma (\alpha)}(\frac{1}{x})^{\alpha+1}e^{-\frac{\beta}{x}}$
I need to find the distribution of $T=X\sqrt{Y}$.
According to my calculations, T is not a non-central Student's t-distribution , it is a non-standardized Student's t instead with $2\alpha$ degrees of freedom, location parameter $\mu$ and a scale parameter $\sqrt{\frac{\beta}{\alpha}}$.
Is it correct?
Thank you.
EDIT:
These are my calculations:
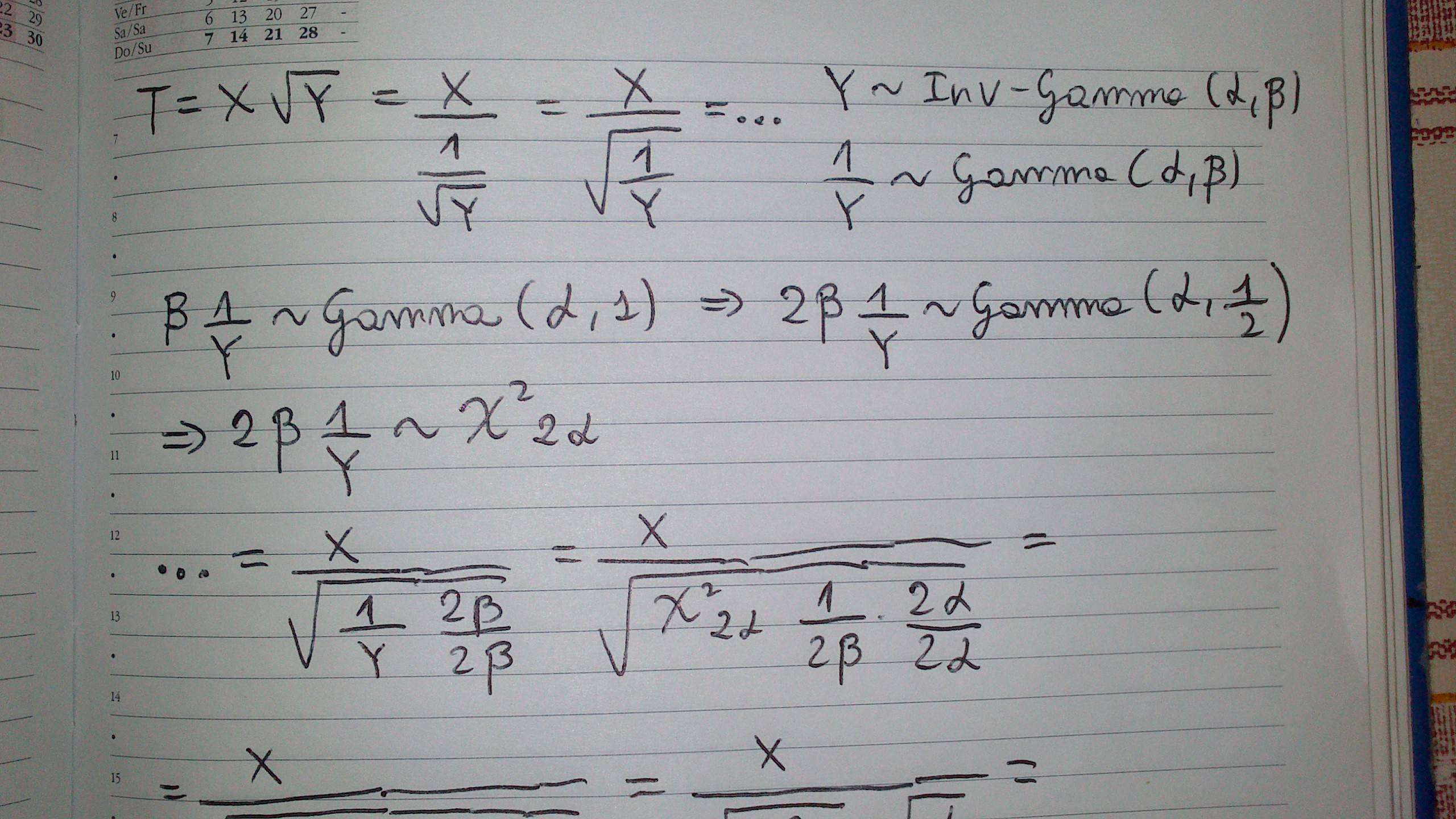
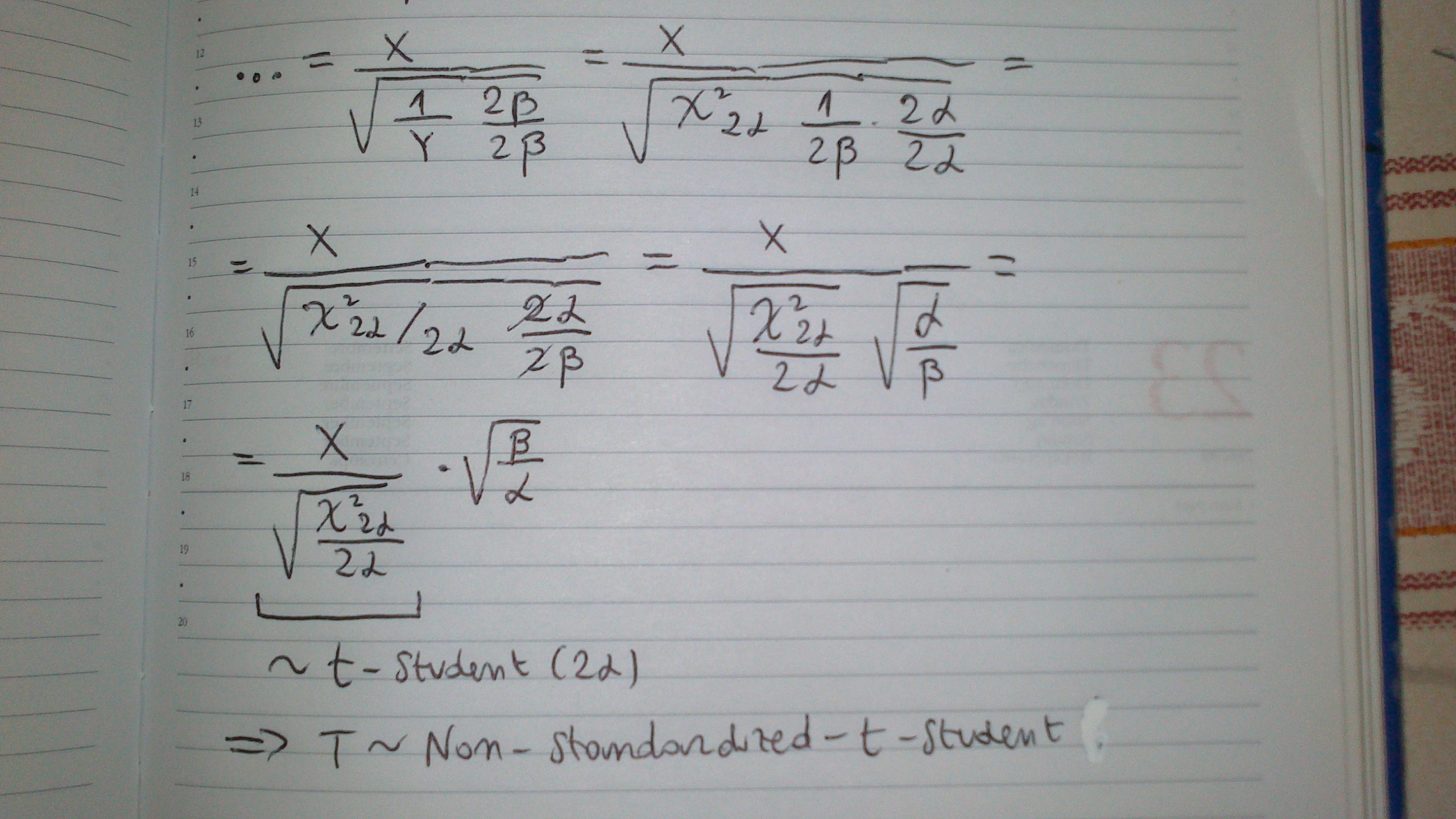

self-studytag (see its tag wiki for the special way such questions may be dealt with). Since you can only have 5 tags, I'd suggest replacing thedistributionstag. Perhaps you could (i) explain why you expect it to be non-central-t (since you raise that possibility), and (ii) show your work (i.e. why you now think it isn't), so that we can give more specific guidance. $\endgroup$