lets suppose a bivariate empirical copula as:
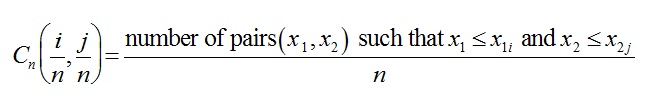
for a set of data of example data we can plot it like this:
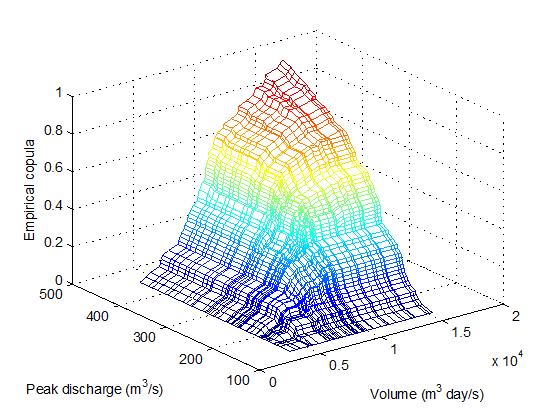
How can we compute the joint cdf of this empirical copula which should like this:
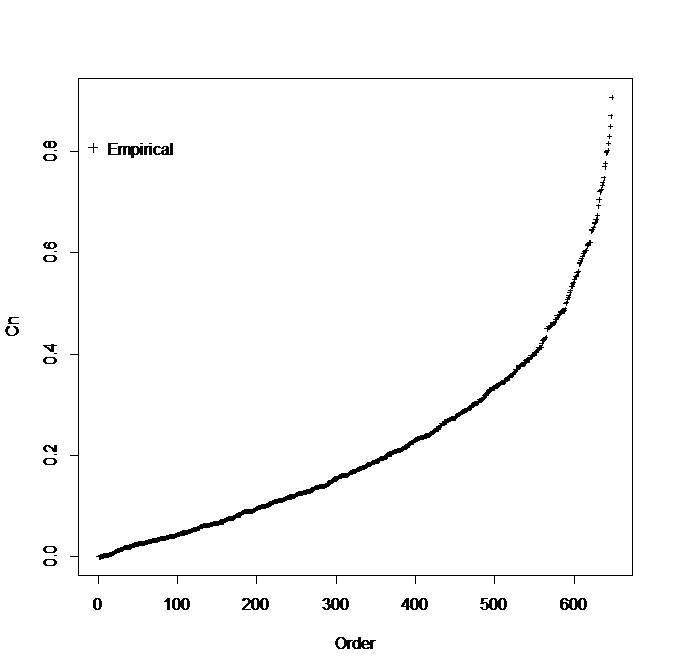 Thank you
Thank you
lets suppose a bivariate empirical copula as:
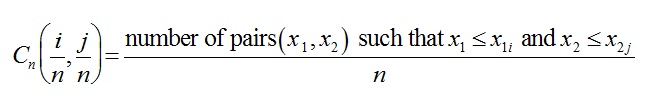
for a set of data of example data we can plot it like this:

How can we compute the joint cdf of this empirical copula which should like this:
 Thank you
Thank you
Your empirical CDF copula has support in [0,1]^3 (I guess your observations are n ordered triplets). So, I don´t understand your graph: what do you mean by "order" in the horizontal axis?