Problem:
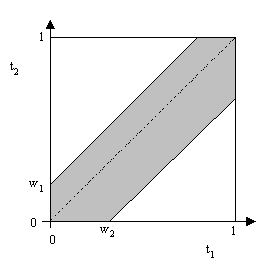
Two people are to arrive at the same location some time between 3pm and 4pm. They both arrive at random times within the hour and only stay for ten minutes. Since they only go one time, what is the probability that they will meet during that hour?
I have found the correct answer to this problem here (the second formula P2): http://www.mathpages.com/home/kmath124/kmath124.htm
$$ P2 = 1 - \frac{(1-w_1)^2 + (1-w_2)^2}{2} = w_1 + w_2 - \frac{w_1^2}{2} - \frac{w_2^2}{2} $$
Plugging in ($1/6$) for both $w_1$ and $w_2$ is $11/36$ which is the correct answer.
But I don't understand how they came to that conclusion and what formula they derived it from. Any help in explaining this would be appreciated!