Two easy probability tasks

Question

I'm struggling with two exercises for which I do possess answers, but I have no idea why they would be like that. I haven't done any statistics in a long time (restarting studies).

Question 1

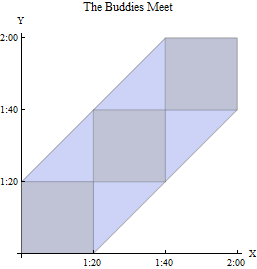
Two buddies agreed to come to a meeting between 1 pm and 2 pm. Each one will come at random time and will be waiting for 20 minutes before going back home. What's the chance for them to meet?

I have an answer written down as 5/9 (to be more specific, (3600-1600)/3600)
It seems to be done by contradiction, but I haven't yet come to a conclusion what kind.

Question 2

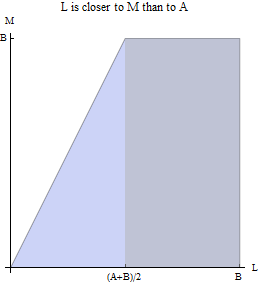
Over a section $AB$ which has a length of $l$, two points $M$ and $L$ were chosen randomly. Find the probability of $L$ being closer to the $M$ than $A$.

So what I have is something like this:
$$ A-------L-------M-------B $$ where $|AL| = x$ and $|AM| = y$. I concluded that in order for $L$ to be closer to $M$ than $|AM|$ there must be such that $|y-x|< x$, that is $-x < y-x < x$, so $y>0$ and $y<2x$.
So my question is, how do I get the probability from this equation? (default answer is 3/4)

Answer 1

A quick and easy way to address both questions is to plot the events. Because the distributions of all the variables involved--arrival times of the buddies and locations of the points--are all uniform, the area of the plot (relative to the total area that could be plotted) gives the answer.

1. The Buddies Meet

Let $X$ be the time the first buddy arrives and $Y$ the time the second one does. The condition of their meeting, measured in hours, is

$$X \le Y \le X + 20/60\text{ or } Y \le X \le Y + 20/60.$$

The plot of these points can be seen to comprise three squares, of $20/60=1/3$ hour on a side, plus two sets of diagonal half-squares of the same size. That's the equivalent of five such squares. Each square has $(1/3)^2=1/9$ the area of the whole square covering all possible arrival times $(X,Y)$ between $1$ and $2$ pm, whence the answer is $5/9$.

2. A Point Proximity Problem

This time, in the $(L,M)$ plane, the event is

$$|L-M| \lt |L-A|.$$

Geometrically it comprises a rectangle of base $(B-A)/2$ and height $(B-A)$ adjoined to a diagonal half-rectangle of the same dimensions. Clearly these fill $3/4$ of the area of the square $[A,B]\times [A,B]$.

---

Even in more complicated problems involving non-uniform univariate, bivariate, (or even trivariate) probabilities, drawing pictures of events is often very helpful for understanding how to set up and compute the integrals that must be evaluated.

Answer 2

Since the question is flagged as self-study, I'll just provide some hints to (hopefully) help you derive the solution. I'll amend/complete my answer based on your progress.

Question 1

As a starting point, you might want express the information provided in the problem with some mathematical notation. To do so, define two (continuous) random variables, say $X$ and $Y$, corresponding to the arrival times (in minutes after 1pm) of the two buddies. What are the conditions on $X$ and $Y$ for the two buddies to meet, i.e., what is the domain of values for $X$ and $Y$ where the two buddies meet?

To compute the requested probability, one needs to know the joint distribution of $X$ and $Y$. It is not explicitly provided in the question; I'll assume that $X$ and $Y$ are independant and uniformly distributed on $[0, 60]$.

You have (at least) two options here. The first approach is based on a geometric reasoning and works it only because the variables are independant and uniformly distributed. The requested probability is given by the ratio of the area of domain of $X$ and $Y$ where the two buddies meet and the total area of the domain of possible vaues for $X$ and $Y$.

In the second approach the requested probability is obtained by integrating the joint density on the domain of $X$ and $Y$ corresponding to situations where the two buddies meet.

Answer 3

For question 2 here are some things to consider.

This all assumes that the points will be placed based on a uniform distribution (reasonable but unstated assumption).

Your drawing shows the order A L M B, but from the description it seems that you could also see A M L B, in the later case L will always be closer to M than to A, what is the probability of seeing A M L B and seeing A L M B?

Now conditioning on the order A L M B, think about having placed M (and A is already there) and we know that L will fall between A and M, what proportion of the line will have L closer to A? closer to M?

Combine the answers to the 2 questions and you should see $\frac34$.